Conformal-differential geometry
A branch of conformal geometry in which the geometric quantities that are invariant under conformal transformations are studied by the methods of analysis, in the first instance, differential calculus.
In the conformal plane 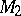 each point or circle is defined by a vector
each point or circle is defined by a vector 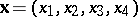 , where the
, where the 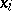 ,
, 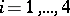 , are the so-called tetracyclic coordinates. For a point one has
, are the so-called tetracyclic coordinates. For a point one has
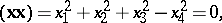 |
and for a circle 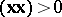 . The conformal-differential geometry in the plane studies sequences and congruences of circles. To a sequence of circles corresponds a curve in three-dimensional hyperbolic space and to a congruence of circles — a surface. A sequence is given by a parametrization
. The conformal-differential geometry in the plane studies sequences and congruences of circles. To a sequence of circles corresponds a curve in three-dimensional hyperbolic space and to a congruence of circles — a surface. A sequence is given by a parametrization 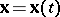 . The parameter
. The parameter  can be specialized to
can be specialized to
 |
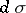 being the angle between two infinitesimally-close circles in the sequence. Of special significance in the theory of sequences are the two branches of the envelope of this sequence,
being the angle between two infinitesimally-close circles in the sequence. Of special significance in the theory of sequences are the two branches of the envelope of this sequence, 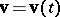 and
and 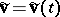 , their osculating circles. As in ordinary differential geometry of curves, one can write derivation formulas for a sequence of circles by decomposing the derivatives of the vectors
, their osculating circles. As in ordinary differential geometry of curves, one can write derivation formulas for a sequence of circles by decomposing the derivatives of the vectors  ,
, 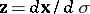 ,
,  ,
, 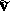 in terms of themselves:
in terms of themselves:
 |
 |
Two invariants 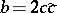 and
and 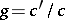 can be obtained. The invariant
can be obtained. The invariant  is expressed in terms of the angle
is expressed in terms of the angle  between the osculating circles of the envelope:
between the osculating circles of the envelope: 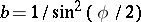 . The theory of curves in the conformal plane is constructed from the theory of sequences of circles: each curve is regarded as an envelope, namely the sequence with invariant
. The theory of curves in the conformal plane is constructed from the theory of sequences of circles: each curve is regarded as an envelope, namely the sequence with invariant 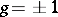 . If, furthermore, the invariant
. If, furthermore, the invariant 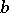 is constant, then the curve turns out to be the isogonal trajectory of a pencil of circles, that is, a loxodrome.
is constant, then the curve turns out to be the isogonal trajectory of a pencil of circles, that is, a loxodrome.
In the three-dimensional space 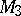 the equation
the equation  defines a sequence of spheres. In its study its enveloping surface, the so-called canal surface, plays an important role. Each sequence of spheres is characterized by three invariants, which are expressed in terms of certain angles determined by the spheres of the sequence.
defines a sequence of spheres. In its study its enveloping surface, the so-called canal surface, plays an important role. Each sequence of spheres is characterized by three invariants, which are expressed in terms of certain angles determined by the spheres of the sequence.
A congruence of circles in 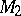 is given by a parametrization
is given by a parametrization 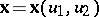 . On the surface corresponding to it in the hyperbolic space, the polar normalization is conveniently introduced by taking as the normal of the first kind the line orthogonal to the tangent plane of the surface at the point
. On the surface corresponding to it in the hyperbolic space, the polar normalization is conveniently introduced by taking as the normal of the first kind the line orthogonal to the tangent plane of the surface at the point  , and as the normal of the second kind, the polar of the normal of the first kind with respect to the absolute
, and as the normal of the second kind, the polar of the normal of the first kind with respect to the absolute  (see [3]). In
(see [3]). In 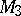 the normalization of a congruence corresponds to the normalization of the surface: Associated with each circle
the normalization of a congruence corresponds to the normalization of the surface: Associated with each circle  is the circle
is the circle 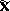 orthogonal to
orthogonal to  and to each infinitesimally-close circle, and two circles
and to each infinitesimally-close circle, and two circles 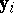 , defining the pencil of the circles, conjugate to the pencil
, defining the pencil of the circles, conjugate to the pencil  :
: 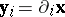 . On
. On 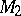 invariants of the congruence of circles are defined corresponding to the invariants of the associated surface in hyperbolic space, and special types of congruences are singled out.
invariants of the congruence of circles are defined corresponding to the invariants of the associated surface in hyperbolic space, and special types of congruences are singled out.
In the theory of surfaces in 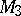 a framing of the surface is introduced by means of normal circles orthogonal at each point to all tangent spheres of the surface; associated with each point is a conformal frame consisting of a point of the surface
a framing of the surface is introduced by means of normal circles orthogonal at each point to all tangent spheres of the surface; associated with each point is a conformal frame consisting of a point of the surface  , two coordinate spheres
, two coordinate spheres 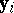 ,
, 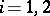 , defining normal circles, a tangent sphere
, defining normal circles, a tangent sphere  at
at  , and the point of intersection
, and the point of intersection 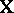 of this sphere and the normal circle. In the general theory of normalization of surfaces one uses the isomorphism of the theory of normalized surfaces of a conformal space and the theory of interior polar normalizations of the absolute of a hyperbolic space. The interior geometry of a normalized surface in
of this sphere and the normal circle. In the general theory of normalization of surfaces one uses the isomorphism of the theory of normalized surfaces of a conformal space and the theory of interior polar normalizations of the absolute of a hyperbolic space. The interior geometry of a normalized surface in 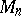 is a Weyl geometry whose first fundamental tensor is the same as the tensor of the angular metric of the surface, while the second fundamental tensor is the normalizer defining the supporting coordinates of the sphere.
is a Weyl geometry whose first fundamental tensor is the same as the tensor of the angular metric of the surface, while the second fundamental tensor is the normalizer defining the supporting coordinates of the sphere.
The results obtained for a normalized surface are valid also for a normalized conformal space.
References
| [1] | G. Darboux, "Leçons sur la théorie générale des surfaces et ses applications géométriques du calcul infinitésimal" , 1–4 , Gauthier-Villars (1887–1896) |
| [2] | W. Blaschke, "Vorlesungen über Differentialgeometrie und geometrische Grundlagen von Einstein's Relativitätstheorie" , 3. Differentialgeometrie der Kreisen und Kugeln , Springer (1929) |
| [3] | A.P. Norden, "Spaces with an affine connection" , Nauka , Moscow-Leningrad (1976) (In Russian) |
| [4] | G.V. Bushmanova, A.P. Norden, "Elements of conformal geometry" , Kazan' (1972) (In Russian) |
Conformal-differential geometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conformal-differential_geometry&oldid=12839