Cobordism of knots
knot cobordism (proper bordism of knots, see Bordism)
An equivalence relation on the set of knots, weaker than the isotopy type relation. Two smooth  -dimensional knots
-dimensional knots  and
and 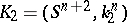 are said to be cobordant if there exists a smooth oriented
are said to be cobordant if there exists a smooth oriented  -dimensional submanifold
-dimensional submanifold 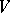 of
of 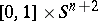 , where
, where 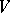 is homeomorphic to
is homeomorphic to  and
and 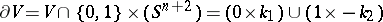 . Here the minus sign indicates the opposite orientation. Knots cobordant to the trivial knot are called cobordant to zero, or slice knots. The set of (cobordance) equivalence classes of
. Here the minus sign indicates the opposite orientation. Knots cobordant to the trivial knot are called cobordant to zero, or slice knots. The set of (cobordance) equivalence classes of  -dimensional smooth knots is denoted by
-dimensional smooth knots is denoted by 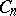 . The operation of connected sum defines on
. The operation of connected sum defines on 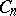 an Abelian group structure. The inverse of the knot cobordism class
an Abelian group structure. The inverse of the knot cobordism class 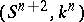 is the knot cobordism class
is the knot cobordism class  .
.
For every even  the group
the group 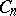 is zero. The knot cobordism class of an odd-dimensional knot is defined by its Seifert matrix. A square integral matrix
is zero. The knot cobordism class of an odd-dimensional knot is defined by its Seifert matrix. A square integral matrix 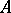 is called cobordant to zero if it is unimodularly congruent to a matrix of the form
is called cobordant to zero if it is unimodularly congruent to a matrix of the form
 |
where 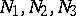 are square matrices of the same dimensions and 0 is the zero matrix. Two square matrices
are square matrices of the same dimensions and 0 is the zero matrix. Two square matrices 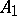 and
and 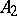 are called cobordant if the matrix
are called cobordant if the matrix
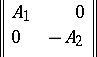 |
is cobordant to zero. A square integral matrix 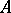 is called an
is called an  -matrix, where
-matrix, where 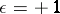 or
or 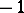 , if
, if  . The Seifert matrix of every
. The Seifert matrix of every 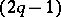 -dimensional knot is a
-dimensional knot is a 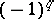 -matrix. For every
-matrix. For every  the cobordance relation is an equivalence relation on the set of all
the cobordance relation is an equivalence relation on the set of all  -matrices. The set of equivalence classes is denoted by
-matrices. The set of equivalence classes is denoted by 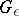 . The operation of direct sum defines on
. The operation of direct sum defines on 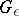 an Abelian group structure. One has the Levine homomorphism
an Abelian group structure. One has the Levine homomorphism 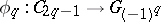 which associates with the cobordism class of the knot
which associates with the cobordism class of the knot 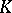 the cobordism class of the Seifert matrix of
the cobordism class of the Seifert matrix of 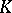 . The Levine homomorphism is an isomorphism for all
. The Levine homomorphism is an isomorphism for all 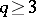 . The homomorphism
. The homomorphism 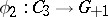 is a monomorphism, its image is a subgroup of index 2 in
is a monomorphism, its image is a subgroup of index 2 in 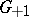 , consisting of the class of
, consisting of the class of 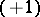 -matrices
-matrices 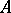 for which the signature of
for which the signature of  is divisible by 16. The homomorphism
is divisible by 16. The homomorphism 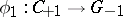 is an epimorphism; its kernel is non-trivial.
is an epimorphism; its kernel is non-trivial.
For a study of the structure of the groups 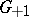 and
and 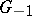 and for the construction of a complete system of invariants of knot cobordism classes one makes use of the following construction. An isometric structure over a field
and for the construction of a complete system of invariants of knot cobordism classes one makes use of the following construction. An isometric structure over a field 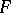 is a pair
is a pair 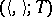 consisting of a non-degenerate quadratic form
consisting of a non-degenerate quadratic form 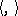 given on a finite-dimensional vector space
given on a finite-dimensional vector space 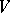 over
over 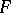 and an isometry
and an isometry 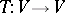 . An isometric structure
. An isometric structure 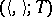 is called cobordant to zero if
is called cobordant to zero if 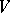 contains a totally-isotropic subspace of half its dimension that is invariant under
contains a totally-isotropic subspace of half its dimension that is invariant under 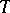 . The operation of orthogonal sum of forms and direct sum of isometries defines an operation
. The operation of orthogonal sum of forms and direct sum of isometries defines an operation  on the set of isometric structures. Two isometric structures
on the set of isometric structures. Two isometric structures 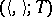 and
and 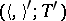 are called cobordant if the isometric structure
are called cobordant if the isometric structure  is cobordant to zero. Let
is cobordant to zero. Let  be the set of cobordism classes of isometric structures
be the set of cobordism classes of isometric structures 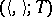 satisfying the condition
satisfying the condition 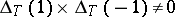 , where
, where 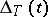 is the characteristic polynomial of the isometry
is the characteristic polynomial of the isometry 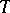 . In the study of the groups
. In the study of the groups 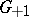 and
and 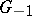 an important role is played by the imbeddings
an important role is played by the imbeddings 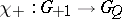 and
and 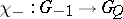 , which are constructed as follows. Every cobordism class of
, which are constructed as follows. Every cobordism class of  -matrices contains a non-degenerate matrix. If
-matrices contains a non-degenerate matrix. If 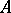 is a non-degenerate
is a non-degenerate  -matrix, put
-matrix, put 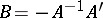 ,
, 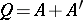 and let
and let 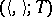 be the isometric structure whose form
be the isometric structure whose form 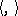 has the given matrix
has the given matrix 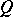 , while its isometry
, while its isometry 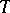 has the matrix
has the matrix 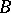 . This gives a well-defined homomorphism
. This gives a well-defined homomorphism 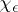 with
with 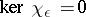 .
.
Let 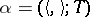 be an isometric structure on a vector space
be an isometric structure on a vector space 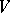 and let
and let 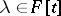 . Denote by
. Denote by 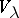 the
the 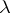 -primary component of
-primary component of 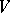 , i.e.
, i.e. 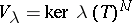 for large
for large 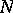 . A polynomial
. A polynomial 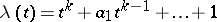 is called reciprocal if
is called reciprocal if  for all
for all 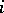 . For each irreducible reciprocal polynomial
. For each irreducible reciprocal polynomial 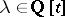 denote by
denote by 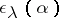 the exponent, reduced modulo 2, with which
the exponent, reduced modulo 2, with which 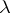 divides the characteristic polynomial
divides the characteristic polynomial 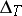 of the isometry
of the isometry 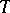 . For every reciprocal polynomial
. For every reciprocal polynomial 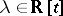 irreducible over
irreducible over 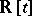 , denote by
, denote by 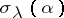 the signature of the restriction of
the signature of the restriction of 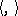 to
to 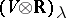 . For each prime number
. For each prime number 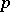 and each reciprocal polynomial
and each reciprocal polynomial 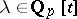 irreducible over
irreducible over  , denote by
, denote by  the restriction of
the restriction of  to
to 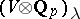 , where
, where  is the field of
is the field of 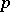 -adic numbers. Put
-adic numbers. Put
 |
where  is the Hilbert symbol in
is the Hilbert symbol in 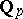 ,
,  is the Hasse symbol and
is the Hasse symbol and 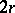 is the rank of
is the rank of 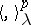 . Two isometric structures
. Two isometric structures 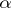 and
and 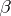 are cobordant if and only if
are cobordant if and only if  ,
,  and
and 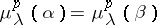 for all
for all  and
and 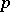 for which these invariants are defined (cf. [3], [4]).
for which these invariants are defined (cf. [3], [4]).
The compositions of the Levine homomorphism, the homomorphism 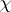 and the functions
and the functions 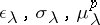 associate with every odd-dimensional knot
associate with every odd-dimensional knot 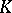 the numbers
the numbers 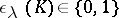 ,
,  ,
, 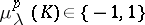 . Two
. Two 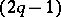 -dimensional knots
-dimensional knots 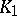 and
and 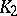 , where
, where 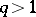 , are cobordant if and only if
, are cobordant if and only if
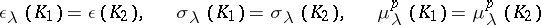 |
for all  and
and 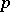 for which these invariants are defined.
for which these invariants are defined.  is equal to the signature of the knot
is equal to the signature of the knot 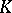 (cf. Knots and links, quadratic forms of), where the sum is extended over all
(cf. Knots and links, quadratic forms of), where the sum is extended over all 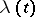 of the form
of the form 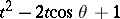 , where
, where 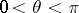 , and in the sum only a finite number of terms are distinct from zero.
, and in the sum only a finite number of terms are distinct from zero.
Similarly one defines the group of locally flat or piecewise-linear knot cobordisms, denoted by 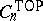 and
and 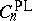 , respectively. For all
, respectively. For all  one has an isomorphism
one has an isomorphism 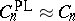 . The natural mapping
. The natural mapping 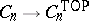 is an isomorphism for
is an isomorphism for 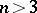 , while for
, while for 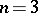 it is a monomorphism with an image of index 2. This means, in particular, that there exists a non-smooth locally flat topologically three-dimensional knot in
it is a monomorphism with an image of index 2. This means, in particular, that there exists a non-smooth locally flat topologically three-dimensional knot in 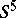 (cf. [5]).
(cf. [5]).
The theory of cobordism of knots is connected with the study of singularities of not locally flat or piecewise-linear imbeddings of codimension 2. If 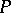 is an
is an 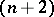 -dimensional oriented manifold, imbedded as a subcomplex in an
-dimensional oriented manifold, imbedded as a subcomplex in an 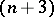 -dimensional manifold
-dimensional manifold 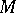 ,
, 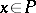 , and
, and 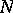 is a small star-shaped neighbourhood of
is a small star-shaped neighbourhood of  in
in 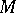 , then the singularity of the imbedding of
, then the singularity of the imbedding of  in
in  at
at  may be measured as follows. The boundary
may be measured as follows. The boundary  is an
is an 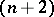 -dimensional sphere, the orientation of which is defined by that of
-dimensional sphere, the orientation of which is defined by that of 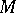 ;
; 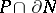 is an
is an  -dimensional sphere the orientation of which is defined by that of
-dimensional sphere the orientation of which is defined by that of 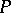 . This defines an
. This defines an  -dimensional knot
-dimensional knot 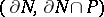 , called the singularity of the imbedding
, called the singularity of the imbedding  at the point
at the point  .
.
References
| [1] | R.H. Fox, J.W. Milnor, "Singularities of 2-spheres in 4-space and cobordism of knots" Osaka Math. J. , 3 (1966) pp. 257–267 |
| [2] | M.A. Kervaire, "Les noeuds de dimensions supérieures" Bull. Soc. Math. France , 93 (1965) pp. 225–271 |
| [3] | J. Levine, "Knot cobordism groups in codimension 2" Comment. Math. Helv. , 44 (1969) pp. 229–244 |
| [4] | J. Levine, "Invariants of knot cobordism" Invent. Math. , 8 (1969) pp. 98–110 |
| [5] | S.E. Capell, J.L. Shaneson, "Topological knots and knot cobordism" Topology , 12 (1973) pp. 33–40 |
| [6] | N.W. Stoltzfus, "Unraveling the integral knot concordance group" Mem. Amer. Math. Soc. , 12 (1977) pp. 192 |
Comments
Another term for cobordance of knots is concordance of knots, and correspondingly one has the knot concordance group.
References
| [a1] | L.H. Kaufmann, "On knots" , Princeton Univ. Press (1987) |
Cobordism of knots. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cobordism_of_knots&oldid=14400