Difference between revisions of "Chi-squared distribution"
(Importing text file) |
m (moved "Chi-squared" distribution to Chi-squared distribution: Modify alphabetical position so this item will appear in "C" rather than before "A".) |
(No difference)
| |
Revision as of 20:31, 27 March 2011
 -distribution
-distribution
The continuous probability distribution, concentrated on the positive semi-axis  , with density
, with density
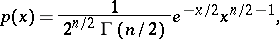 |
where  is the gamma-function and the positive integral parameter
is the gamma-function and the positive integral parameter  is called the number of degrees of freedom. A "chi-squared" distribution is a special case of a gamma-distribution and has all the properties of the latter. The distribution function of a "chi-squared" distribution is an incomplete gamma-function, the characteristic function is expressed by the formula
is called the number of degrees of freedom. A "chi-squared" distribution is a special case of a gamma-distribution and has all the properties of the latter. The distribution function of a "chi-squared" distribution is an incomplete gamma-function, the characteristic function is expressed by the formula
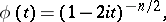 |
and the mathematical expectation and variance are  and
and  , respectively. The family of "chi-squared" distributions is closed under the operation of convolution.
, respectively. The family of "chi-squared" distributions is closed under the operation of convolution.
The "chi-squared" distribution with  degrees of freedom can be derived as the distribution of the sum
degrees of freedom can be derived as the distribution of the sum  of the squares of independent random variables
of the squares of independent random variables 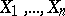 having identical normal distributions with mathematical expectation 0 and variance 1. This connection with a normal distribution determines the role that the "chi-squared" distribution plays in probability theory and in mathematical statistics.
having identical normal distributions with mathematical expectation 0 and variance 1. This connection with a normal distribution determines the role that the "chi-squared" distribution plays in probability theory and in mathematical statistics.
Many distributions can be defined by means of the "chi-squared" distribution. For example, the distribution of the random variable 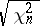 — the length of the random vector
— the length of the random vector  with independent normally-distributed components — (sometimes called a "chi" -distribution, see also the special cases of a Maxwell distribution and a Rayleigh distribution), the Student distribution, and the Fisher
with independent normally-distributed components — (sometimes called a "chi" -distribution, see also the special cases of a Maxwell distribution and a Rayleigh distribution), the Student distribution, and the Fisher 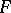 -distribution. In mathematical statistics these distributions together with the "chi-squared" distribution describe sample distributions of various statistics of normally-distributed results of observations and are used to construct statistical interval estimators and statistical tests. A special reputation in connection with the "chi-squared" distribution has been gained by the "chi-squared" test, based on the so-called "chi-squared" statistic of E.S. Pearson.
-distribution. In mathematical statistics these distributions together with the "chi-squared" distribution describe sample distributions of various statistics of normally-distributed results of observations and are used to construct statistical interval estimators and statistical tests. A special reputation in connection with the "chi-squared" distribution has been gained by the "chi-squared" test, based on the so-called "chi-squared" statistic of E.S. Pearson.
There are detailed tables of the "chi-squared" distribution which are convenient for statistical calculations. For large  one uses approximations by means of a normal distribution; for example, according to the central limit theorem, the distribution of the normalized variable
one uses approximations by means of a normal distribution; for example, according to the central limit theorem, the distribution of the normalized variable  converges to the standard normal distribution. More accurate is the approximation
converges to the standard normal distribution. More accurate is the approximation
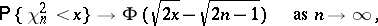 |
where  is the standard normal distribution function.
is the standard normal distribution function.
See also Non-central "chi-squared" distribution.
References
| [1] | H. Cramér, "Mathematical methods of statistics" , Princeton Univ. Press (1946) |
| [2] | M.G. Kendall, A. Stuart, "The advanced theory of statistics. Distribution theory" , 1 , Griffin (1969) |
| [3] | H.O. Lancaster, "The chi-squared distribution" , Wiley (1969) |
| [4] | L.N. Bol'shev, N.V. Smirnov, "Tables of mathematical statistics" , Libr. math. tables , 46 , Nauka (1983) (In Russian) (Processed by L.S. Bark and E.S. Kedrova) |
Comments
The name "chi-square" distribution is also used.
Chi-squared distribution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Chi-squared_distribution&oldid=19334