Branch point
singular point of multi-valued character
An isolated singular point  of an analytic function
of an analytic function  of one complex variable
of one complex variable  such that the analytic continuation of an arbitrary function element of
such that the analytic continuation of an arbitrary function element of 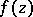 along a closed path which encircles
along a closed path which encircles  yields new elements of
yields new elements of  . More exactly,
. More exactly,  is said to be a branch point if there exist: 1) an annulus
is said to be a branch point if there exist: 1) an annulus 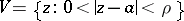 in which
in which 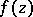 can be analytically extended along any path; 2) a point
can be analytically extended along any path; 2) a point  and some function element of
and some function element of 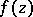 represented by a power series
represented by a power series
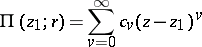 |
with centre 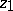 and radius of convergence
and radius of convergence 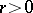 , the analytic continuation of which along the circle
, the analytic continuation of which along the circle 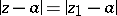 , going around the path once in, say, the positive direction, yields a new element
, going around the path once in, say, the positive direction, yields a new element  different from
different from 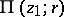 . If, after a minimum number
. If, after a minimum number 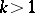 of such rounds the initial element
of such rounds the initial element 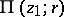 is again obtained, this is also true of all elements of the branch (cf. Branch of an analytic function) of
is again obtained, this is also true of all elements of the branch (cf. Branch of an analytic function) of 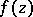 defined in
defined in  by the element
by the element  . In such a case
. In such a case  is a branch point of finite order
is a branch point of finite order 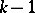 of this branch. In a punctured neighbourhood
of this branch. In a punctured neighbourhood  of a branch point
of a branch point  of finite order this branch is represented by a generalized Laurent series, or Puiseux series:
of finite order this branch is represented by a generalized Laurent series, or Puiseux series:
 | (1) |
If 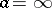 is an improper branch point of a finite order, then the branch of
is an improper branch point of a finite order, then the branch of 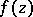 is representable in some neighbourhood
is representable in some neighbourhood 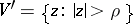 by an analogue of the series (1):
by an analogue of the series (1):
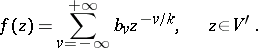 | (2) |
The behaviour of the Riemann surface  of
of  over a branch point of finite order
over a branch point of finite order  is characterized by the fact that
is characterized by the fact that 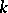 sheets of the branch of
sheets of the branch of 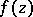 defined by the element
defined by the element  come together over
come together over  . At the same time the behaviour of other branches of
. At the same time the behaviour of other branches of 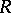 over
over  may be altogether different.
may be altogether different.
If the series (1) or (2) contains only a finite number of non-zero coefficients 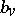 with negative indices
with negative indices  ,
,  is an algebraic branch point or an algebraic singular point. Such a branch point of finite order is also characterized by the fact that as
is an algebraic branch point or an algebraic singular point. Such a branch point of finite order is also characterized by the fact that as  in whatever manner, the values of all elements of the branch defined by
in whatever manner, the values of all elements of the branch defined by 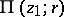 in
in 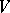 or
or 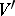 tend to a definite finite or infinite limit.
tend to a definite finite or infinite limit.
Example:  , where
, where  is a natural number,
is a natural number, 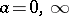 .
.
If the series (1) or (2) contain an infinite number of non-zero coefficients 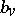 with negative indices
with negative indices  , the branch points of finite order
, the branch points of finite order  belong the class of transcendental branch points.
belong the class of transcendental branch points.
Example: 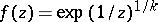 , where
, where 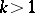 is a natural number,
is a natural number, 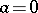 .
.
Finally, if it is impossible to return to the initial element after a finite number of turns,  is said to be a logarithmic branch point or a branch point of infinite order, and is also a transcendental branch point.
is said to be a logarithmic branch point or a branch point of infinite order, and is also a transcendental branch point.
Example: 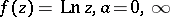 .
.
Infinitely many sheets of the branch of  defined by the element
defined by the element 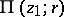 come together over a logarithmic branch point.
come together over a logarithmic branch point.
In the case of an analytic function of several complex variables 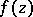 ,
, 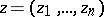 ,
,  , a point
, a point  of the space
of the space  or
or 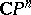 is said to be a branch point of order
is said to be a branch point of order  ,
,  , if it is a branch point of order
, if it is a branch point of order  of the, generally many-sheeted, domain of holomorphy of
of the, generally many-sheeted, domain of holomorphy of 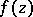 . Unlike in the case
. Unlike in the case  , branch points, just like other singular points of analytic functions (cf. Singular point), cannot be isolated if
, branch points, just like other singular points of analytic functions (cf. Singular point), cannot be isolated if  .
.
References
| [1] | A.I. Markushevich, "Theory of functions of a complex variable" , 2 , Chelsea (1977) pp. Chapt. 8 (Translated from Russian) |
| [2] | B.A. Fuks, "Theory of analytic functions of several complex variables" , Amer. Math. Soc. (1963) (Translated from Russian) |
Branch point. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Branch_point&oldid=46148