Bimodule
double module
An Abelian group  that is a left module over a ring
that is a left module over a ring  and a right module over a ring
and a right module over a ring  , and is such that
, and is such that  for all
for all  ,
,  ,
,  . One says that this is the situation
. One says that this is the situation  , or that
, or that  is an
is an 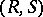 -bimodule. The bimodule
-bimodule. The bimodule 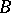 may be regarded as a left
may be regarded as a left 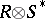 -module, where
-module, where 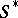 is the ring which is dually isomorphic (anti-isomorphic) to
is the ring which is dually isomorphic (anti-isomorphic) to 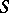 , while
, while  denotes the tensor product over the ring of integers, and
denotes the tensor product over the ring of integers, and 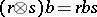 . For every left
. For every left  -module
-module 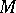 one has the situation
one has the situation  , where
, where 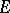 is the ring of endomorphisms of
is the ring of endomorphisms of 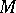 . Any ring
. Any ring  can be given the natural structure of an
can be given the natural structure of an 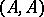 -bimodule.
-bimodule.
Comments
A bimodule morphism is a mapping from a bimodule  into a bimodule
into a bimodule 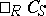 that is left
that is left 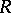 -linear and right
-linear and right 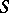 -linear. The category of
-linear. The category of 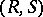 -bimodules with bimodule morphisms is a Grothendieck category.
-bimodules with bimodule morphisms is a Grothendieck category.
The centre of an 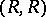 -bimodule (also called an
-bimodule (also called an 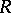 -bimodule)
-bimodule) 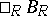 is defined to be the set
is defined to be the set 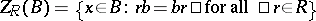 . Clearly
. Clearly 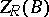 is a two-sided
is a two-sided  -module.
-module.
Bimodule. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bimodule&oldid=13998