Besicovitch almost-periodic functions
A class of almost-periodic functions in which the analogue of the Riesz–Fischer theorem is valid: Any trigonometric series
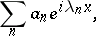 |
where
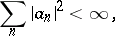 |
is the Fourier series of some 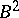 -almost-periodic function. The definition of these functions [1], [2] is based on a generalization of the concept of an almost-period, and certain additional ideas must be introduced in it. A set
-almost-periodic function. The definition of these functions [1], [2] is based on a generalization of the concept of an almost-period, and certain additional ideas must be introduced in it. A set 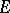 of real numbers is called sufficiently homogeneous if there exists an
of real numbers is called sufficiently homogeneous if there exists an 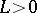 such that the ratio between the largest number of members of
such that the ratio between the largest number of members of 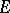 in an interval of length
in an interval of length 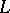 and the smallest number of members in an interval of the same length
and the smallest number of members in an interval of the same length 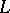 is less than 2. A sufficiently homogeneous set is also relatively dense. A complex-valued function
is less than 2. A sufficiently homogeneous set is also relatively dense. A complex-valued function 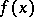 ,
, 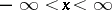 , summable to degree
, summable to degree 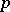 on any finite interval of the real axis, is called a Besicovitch almost-periodic function if to each
on any finite interval of the real axis, is called a Besicovitch almost-periodic function if to each 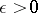 there corresponds a sufficiently homogeneous set of numbers (the so-called
there corresponds a sufficiently homogeneous set of numbers (the so-called 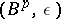 -almost-periods of
-almost-periods of 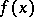 ):
):
 |
such that for each 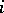
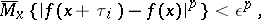 |
and for each 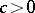
 |
where
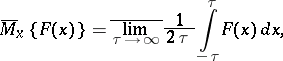 |
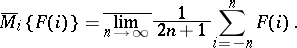 |
Here 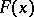 is a real-valued function, defined, respectively, for a real variable and an integer argument.
is a real-valued function, defined, respectively, for a real variable and an integer argument.
References
| [1] | A.S. Besicovitch, "On mean values of functions of a complex and of a real variable" Proc. London Math. Soc. (2) , 27 (1927) pp. 373–388 |
| [2] | A.S. Besicovitch, "On Parseval's theorem for Dirichlet series" Proc. London Math. Soc. (2) , 26 (1927) pp. 25–34 |
| [3] | B.M. Levitan, "Almost-periodic functions" , Moscow (1953) (In Russian) |
Comments
Besicovitch developed his theory in [a1], rather than in [1], [2].
As is implicit in the article, for each 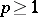 there is a class of almost-periodic functions, denoted by
there is a class of almost-periodic functions, denoted by 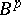 . The first part of the article deals with
. The first part of the article deals with  , the rest is more general. General references may be found under Almost-periodic function.
, the rest is more general. General references may be found under Almost-periodic function.
References
| [a1] | A.S. Besicovitch, "On generalized almost periodic functions" Proc. London Math. Soc. (2) , 25 (1926) pp. 495–512 |
Besicovitch almost-periodic functions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Besicovitch_almost-periodic_functions&oldid=11650