Attainable subgroup
A subgroup  that can be included in a finite normal series of a group
that can be included in a finite normal series of a group 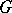 , i.e. in a series
, i.e. in a series
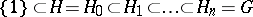 |
in which each subgroup 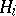 is a normal subgroup in
is a normal subgroup in 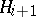 . The property of a subgroup to be attainable is transitive. An intersection of attainable subgroups is an attainable subgroup. The subgroup generated by two attainable subgroups need not be an attainable subgroup. A group
. The property of a subgroup to be attainable is transitive. An intersection of attainable subgroups is an attainable subgroup. The subgroup generated by two attainable subgroups need not be an attainable subgroup. A group  all subgroups of which are attainable satisfies the normalizer condition, i.e. all subgroups differ from their normalizers (cf. Normalizer of a subset). Such a group is therefore locally nilpotent.
all subgroups of which are attainable satisfies the normalizer condition, i.e. all subgroups differ from their normalizers (cf. Normalizer of a subset). Such a group is therefore locally nilpotent.
References
| [1] | A.G. Kurosh, "The theory of groups" , 1–2 , Chelsea (1955–1956) (Translated from Russian) |
Comments
Instead of attainable subgroup, the term accessible subgroup is used in [1]. In the Western literature the term subnormal subgroup is standard for this kind of subgroup.
References
| [a1] | M. Suzuki, "Group theory" , 2 , Springer (1986) |
Attainable subgroup. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Attainable_subgroup&oldid=18484