Associated function
From Encyclopedia of Mathematics
of a complex variable
A function which is obtained in some manner from a given function  with the aid of some fixed function
with the aid of some fixed function  . For example, if
. For example, if
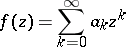 |
is an entire function and if
 |
is a fixed entire function with  ,
,  , then
, then
 |
is a function which is associated to  by means of the function
by means of the function 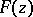 ; it is assumed that the series converges in some neighbourhood
; it is assumed that the series converges in some neighbourhood 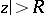 . The function
. The function 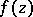 is then represented in terms of
is then represented in terms of 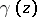 by the formula
by the formula
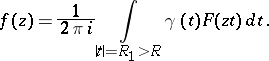 |
In particular, if
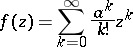 |
is an entire function of exponential type and  , then
, then
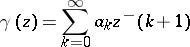 |
is the Borel-associated function of  (cf. Borel transform).
(cf. Borel transform).
How to Cite This Entry:
Associated function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Associated_function&oldid=18474
Associated function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Associated_function&oldid=18474
This article was adapted from an original article by A.F. Leont'ev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article