Appell transformation
A transformation of the equations of plane motion of a material point under the action of a force that varies with the coordinates 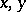 of the point only. The Appell transformation is a homographic transformation of the coordinates
of the point only. The Appell transformation is a homographic transformation of the coordinates 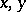 into the coordinates
into the coordinates 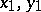 :
:
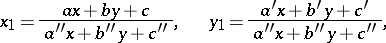 |
which involves the substitution of the time 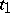 for
for  in accordance with the formula
in accordance with the formula
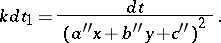 |
As a result of the Appell transformation, the motion of a point 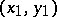 , acted upon by a force
, acted upon by a force 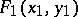 that depends only on the coordinates
that depends only on the coordinates 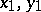 , corresponds to the motion of the point
, corresponds to the motion of the point 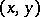 acted upon by the force
acted upon by the force 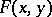 . A transformation of the equations of motion of a point
. A transformation of the equations of motion of a point 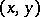 with the aid of formulas of general form
with the aid of formulas of general form
 | (*) |
yields the equations of motion of the point 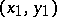 under the effect of a force that depends only on the coordinates
under the effect of a force that depends only on the coordinates  only if (*) is a homographic transformation. The Appell transformation is used to solve Bertrand's problem on the determination of the forces under which the trajectories of motion of material points become conic sections. The Appell transformation is named after P.E. Appell.
only if (*) is a homographic transformation. The Appell transformation is used to solve Bertrand's problem on the determination of the forces under which the trajectories of motion of material points become conic sections. The Appell transformation is named after P.E. Appell.
References
| [1] | P.E. Appell, "De l'homographie en mécanique" Amer. J. Math. , 12 (1890) pp. 103–114 |
| [2] | P.E. Appell, "Sur les lois de forces centrales faisant décrire à leur point d'application une conique quelles que soient les conditions initiales" Amer. J. Math. , 13 (1891) pp. 153–158 |
Appell transformation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Appell_transformation&oldid=12622