Difference between revisions of "Amitsur-Levitzki theorem"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Amitsur–Levitzki theorem to Amitsur-Levitzki theorem: ascii title) |
(No difference)
| |
Revision as of 17:39, 24 March 2012
A basic result in the theory of polynomial identity rings (PI-rings). A ring  is a PI-ring (cf. also PI-algebra) if there is a polynomial in the free associative algebra
is a PI-ring (cf. also PI-algebra) if there is a polynomial in the free associative algebra  which vanishes under all substitutions of ring elements for the variables. The standard polynomial of degree
which vanishes under all substitutions of ring elements for the variables. The standard polynomial of degree  is the polynomial
is the polynomial
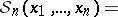 |
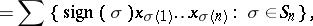 |
where  is the symmetric group on
is the symmetric group on  letters. Since
letters. Since  , a ring is commutative if and only if it satisfies
, a ring is commutative if and only if it satisfies  (cf. also Commutative ring). The Amitsur–Levitzki theorem says that the ring of
(cf. also Commutative ring). The Amitsur–Levitzki theorem says that the ring of  -matrices over a commutative ring satisfies the standard polynomial of degree
-matrices over a commutative ring satisfies the standard polynomial of degree  , and no polynomial of lower degree.
, and no polynomial of lower degree.
There are five different published proofs of this theorem (1996). The original proof by S. Amitsur and J. Levitzki [a1] is a combinatorial argument using matrix units. Because the standard polynomial is linear in each variable, it is enough to prove the theorem when matrix units are substituted for the variables. A streamlined version of this proof can be found in [a3].
The second proof, by B. Kostant [a2], depends upon the Frobenius theory of representations of the alternating group. Kostant's paper was also the first to relate the polynomial identities satisfied by matrices with traces, a theme which was later developed by C. Procesi [a4] and Yu.P. Razmyslov [a5] and influenced much research. The point is that the trace defines a non-degenerate bilinear form on  -matrices.
-matrices.
The third proof, by R.G. Swan [a7], translates the problem into graph theory. The underlying arguments are similar to those in the original proof by Amitsur and Levitzki, but the graph-theoretical approach has led to some generalizations, as in [a8]. The fourth proof, by Razmyslov [a5], is probably the most natural, in that the theorem is deduced in a direct way from the multilinear form of the Cayley–Hamilton theorem. The fifth proof, by S. Rosset [a6], also depends on the Cayley–Hamilton theorem, as well as on elementary properties of the exterior algebra. It is the shortest and most elegant proof, but is not at all straightforward.
References
| [a1] | S.A. Amitsur, J. Levitzki, "Minimal identities for algebras" Proc. Amer. Math. Soc. , 1 (1950) pp. 449–463 |
| [a2] | B. Kostant, "A theorem of Frobenius, a theorem of Amitsur–Levitzki, and cohomology theory" J. Math. Mech. , 7 (1958) pp. 237–264 |
| [a3] | D.S. Passman, "The algebraic structure of group rings" , Wiley (1977) |
| [a4] | C. Procesi, "The invariant theory of  matrices" Adv. in Math. , 19 (1976) pp. 306–381 matrices" Adv. in Math. , 19 (1976) pp. 306–381 |
| [a5] | Yu.P. Razmyslov, "Trace identities of full matrix algebras over a field of characteristic zero" Math. USSR Izv. , 8 (1974) pp. 727–760 Izv. Akad. Nauk SSSR , 38 (1974) pp. 723–756 |
| [a6] | S. Rosset, "A new proof of the Amitsur–Levitzki identity" Israel J. Math. , 23 (1976) pp. 187–188 |
| [a7] | R.G. Swan, "An application of graph theory to algebra" Proc. Amer. Math. Soc. , 14 (1963) pp. 367–380 |
| [a8] | J. Szigeti, Z. Tuza, G. Revesz, "Eulerian polynomial identities on matrix rings" J. Algebra , 161 (1993) pp. 90–101 |
Amitsur-Levitzki theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Amitsur-Levitzki_theorem&oldid=22019