Algebraic surface
A two-dimensional algebraic variety. Together with the class of algebraic curves (cf. Algebraic curve), the class of algebraic surfaces is the class of algebraic varieties which has been most thoroughly studied. The richness of problems and the variety of ideas used to solve them makes the theory of algebraic surfaces one of the most interesting fields of algebraic geometry.
Unlike algebraic curves, two birationally isomorphic algebraic surfaces need not be biregularly isomorphic. During the classical period of the development of algebraic geometry (1868–1920) algebraic surfaces were understood to mean a class of birationally equivalent surfaces, and its representations were considered to be imbedded in complex three-dimensional projective space 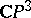 , in which they were defined by a single algebraic equation
, in which they were defined by a single algebraic equation 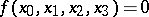 . The foundations of the theory of algebraic surfaces were laid by A. Clebsch and M. Noether (ca. 1870), who defined the first important invariants of algebraic surfaces: the geometric genus and the canonical class. The subsequent development of the theory of algebraic surfaces is due to the Italian school of algebraic geometers: C. Segre, L. Cremona, E. Bertini, G. Castelnuovo, F. Enriques, F. Severi, and others. Topological and transcendental methods were introduced into the theory of algebraic surfaces by E. Picard, H. Poincaré and S. Lefschetz. Many general facts arrived at in these studies proved to be valid for algebraic varieties and schemes of arbitrary dimension as well, while other results up till now have not been generalized to higher-dimensional cases. The latter include the classification of algebraic surfaces by numerical invariants, criteria of rationality and ruledness of algebraic surfaces, and the theory of minimal models. Most of these results were critically reviewed and demonstrated once again in the 1950s and 1960s using modern cohomological methods [1], [5], [8].
. The foundations of the theory of algebraic surfaces were laid by A. Clebsch and M. Noether (ca. 1870), who defined the first important invariants of algebraic surfaces: the geometric genus and the canonical class. The subsequent development of the theory of algebraic surfaces is due to the Italian school of algebraic geometers: C. Segre, L. Cremona, E. Bertini, G. Castelnuovo, F. Enriques, F. Severi, and others. Topological and transcendental methods were introduced into the theory of algebraic surfaces by E. Picard, H. Poincaré and S. Lefschetz. Many general facts arrived at in these studies proved to be valid for algebraic varieties and schemes of arbitrary dimension as well, while other results up till now have not been generalized to higher-dimensional cases. The latter include the classification of algebraic surfaces by numerical invariants, criteria of rationality and ruledness of algebraic surfaces, and the theory of minimal models. Most of these results were critically reviewed and demonstrated once again in the 1950s and 1960s using modern cohomological methods [1], [5], [8].
General features of algebraic surfaces.
Unless otherwise stated, an algebraic surface will be understood to mean a projective algebraic surface over an algebraically closed field 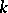 .
.
a) Resolution of singularities. The first rigorous proofs of the existence of a non-singular model were given in the 1930s by R. Walker and O. Zariski. The previous geometrical proofs given by the Italian workers had contained lacunae [9]. The proof of the existence of a non-singular model of an algebraic surface for the case of a ground field with positive characteristic was given in 1956 (cf. Resolution of singularities).
Any non-singular algebraic surface can be birationally projected in  on a surface with ordinary singularities — ordinary double curves with a finite number of ordinary cusp points and triple points, which are triplanar singular points of the surface. Such surfaces represented the principal object of studies during the classical stage of development of the theory of algebraic surfaces. Owing to the theorem on the resolution of singularities of algebraic surfaces, global methods of the theory of schemes may be applied to the local study of singular points of algebraic surfaces. The fundamental work on this subject was done by D. Mumford (1961), who deduced important invariants of two-dimensional singularities and proved that the topological space of a normal algebraic surface is a topological manifold if and only if the surface is non-singular.
on a surface with ordinary singularities — ordinary double curves with a finite number of ordinary cusp points and triple points, which are triplanar singular points of the surface. Such surfaces represented the principal object of studies during the classical stage of development of the theory of algebraic surfaces. Owing to the theorem on the resolution of singularities of algebraic surfaces, global methods of the theory of schemes may be applied to the local study of singular points of algebraic surfaces. The fundamental work on this subject was done by D. Mumford (1961), who deduced important invariants of two-dimensional singularities and proved that the topological space of a normal algebraic surface is a topological manifold if and only if the surface is non-singular.
b) Numerical invariants of algebraic surfaces. The concept of the geometric genus 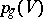 of an algebraic surface is a generalization of the concept of the genus of an algebraic curve in the theory of algebraic surfaces. Here,
of an algebraic surface is a generalization of the concept of the genus of an algebraic curve in the theory of algebraic surfaces. Here, 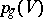 is the maximum number of linearly independent regular two-dimensional differentials on
is the maximum number of linearly independent regular two-dimensional differentials on 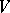 (cf. Geometric genus). The virtual arithmetic genus of the divisors in the canonical system
(cf. Geometric genus). The virtual arithmetic genus of the divisors in the canonical system 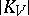 of an algebraic surface
of an algebraic surface 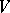 is known as the linear genus and is denoted by
is known as the linear genus and is denoted by  . For a non-singular algebraic surface the following equation is valid:
. For a non-singular algebraic surface the following equation is valid:
 |
where 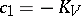 is the first Chern class of the tangent bundle to the surface
is the first Chern class of the tangent bundle to the surface 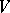 . The multiple canonical systems
. The multiple canonical systems 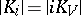 are called pluri-canonical systems of the algebraic surface
are called pluri-canonical systems of the algebraic surface 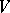 . The number
. The number 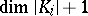 is referred to as the
is referred to as the 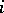 -th (pluri-) genus and is denoted by
-th (pluri-) genus and is denoted by 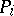 ; it is identical with the dimension of the space of everywhere-regular
; it is identical with the dimension of the space of everywhere-regular 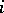 -tuple two-dimensional differential forms on
-tuple two-dimensional differential forms on  .
.
According to the Cayley–Noether postulation formula, the number 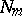 of linearly independent forms of a sufficiently high degree
of linearly independent forms of a sufficiently high degree 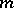 passing through a space curve of degree
passing through a space curve of degree 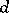 , genus
, genus 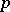 and with
and with 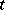 triple points and
triple points and  double points, is given by the formula
double points, is given by the formula
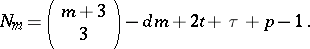 |
The concept of the arithmetic genus 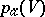 , defined by the formula
, defined by the formula
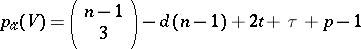 |
(where 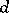 is the degree,
is the degree,  is the number of double points,
is the number of double points, 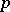 is the genus and
is the genus and  is the number of triple points of the double curve
is the number of triple points of the double curve  ; for non-singular surfaces
; for non-singular surfaces 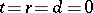 and
and 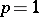 ), was introduced in 1875 for algebraic surfaces with ordinary singularities. Other equivalent definitions of
), was introduced in 1875 for algebraic surfaces with ordinary singularities. Other equivalent definitions of 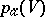 were given subsequently (cf. Arithmetic genus).
were given subsequently (cf. Arithmetic genus).
The difference 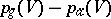 is always non-negative; it is called the irregularity of the algebraic surface
is always non-negative; it is called the irregularity of the algebraic surface  and is denoted by
and is denoted by 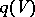 . An algebraic surface is called regular if
. An algebraic surface is called regular if 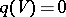 and irregular if
and irregular if 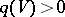 . The first examples of irregular surfaces date back to the end of the 19th century (A. Cayley, Castelnuovo). Irregularity can be described as the maximal insufficiency of the linear system which is cut out by the system
. The first examples of irregular surfaces date back to the end of the 19th century (A. Cayley, Castelnuovo). Irregularity can be described as the maximal insufficiency of the linear system which is cut out by the system 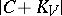 on the curve
on the curve 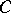 (Enriques, 1896, see [9]). A cohomological definition of
(Enriques, 1896, see [9]). A cohomological definition of 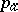 and
and 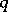 for non-singular algebraic surfaces is given by the formulas:
for non-singular algebraic surfaces is given by the formulas:
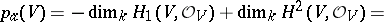 |
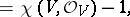 |
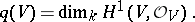 |
The arithmetic genus 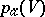 of a non-singular algebraic surface
of a non-singular algebraic surface 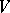 is expressed in terms of the Chern classes of the tangent bundle to
is expressed in terms of the Chern classes of the tangent bundle to 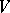 by the formula:
by the formula:
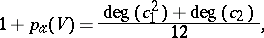 |
called Noether's formula. The number 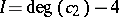 is known as the Zeuten–Segre invariant.
is known as the Zeuten–Segre invariant.
c) The Riemann–Roch theorem for algebraic surfaces. The generalization of the Riemann–Roch theorem for algebraic curves to algebraic surfaces is due to Castelnuovo (1897). For a non-singular algebraic surface  and a complete linear system
and a complete linear system 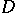 of divisors of virtual degree
of divisors of virtual degree 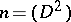 and virtual genus
and virtual genus 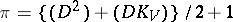 , the Riemann–Roch theorem asserts the inequality
, the Riemann–Roch theorem asserts the inequality
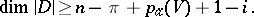 |
The number 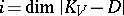 is known as the speciality index of the divisor
is known as the speciality index of the divisor  . The cohomological proof of this theorem (cf. Riemann–Roch theorem) permits the stipulation of a necessary and sufficient condition such that this inequality becomes an equality. This condition is the vanishing of the cohomology group
. The cohomological proof of this theorem (cf. Riemann–Roch theorem) permits the stipulation of a necessary and sufficient condition such that this inequality becomes an equality. This condition is the vanishing of the cohomology group 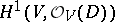 . It has been proved that, for any divisor
. It has been proved that, for any divisor 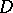 and all sufficiently large numbers
and all sufficiently large numbers  , one has
, one has 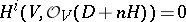 , where
, where 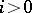 and where
and where 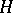 is a hyperplane section of the surface
is a hyperplane section of the surface 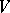 (J.P. Serre, 1955). If the characteristic of the ground field is zero, then the inequality becomes an equality for any ample divisor
(J.P. Serre, 1955). If the characteristic of the ground field is zero, then the inequality becomes an equality for any ample divisor 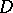 . This result is a generalization of the classical Picard–Severi theorem concerning the regularity of the adjoint system [9].
. This result is a generalization of the classical Picard–Severi theorem concerning the regularity of the adjoint system [9].
d) Systems of curves on an algebraic surface. The principal method used by Italian geometers was their theory of linear and algebraic systems of curves on an algebraic surface. The concepts of algebraic and linear equivalence of divisors are closely connected with this theory. These concepts only coincide for regular algebraic surfaces (Castelnuovo, 1896). Any sufficiently general curve on an algebraic surface 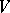 with irregularity
with irregularity 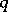 is contained in a maximal algebraic family which fibrates into linear systems of equal dimension, the base of the fibration being a variety of dimension
is contained in a maximal algebraic family which fibrates into linear systems of equal dimension, the base of the fibration being a variety of dimension 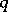 (Enriques, 1904). This variety is an Abelian variety, and is known as the Picard variety of the algebraic surface
(Enriques, 1904). This variety is an Abelian variety, and is known as the Picard variety of the algebraic surface  (cf. Picard scheme). The proof of Enriques' result (known as the "fundamental theorem in the theory of irregular surfacesfundamental theorem in the theory of irregular surfaces" , or the "theorem of completeness of characteristic seriestheorem of completeness of characteristic series" ), as well as subsequent proofs by Severi, contain lacunae. The first rigorous transcendental proof of this theorem was given by Poincaré in 1910, and the algebraic proof came 50 years later (A. Grothendieck, see [5]). If the characteristic of the ground field is positive, this theorem is not valid in the general case (J. Igusa, 1955). It has been shown that, in the general case,
(cf. Picard scheme). The proof of Enriques' result (known as the "fundamental theorem in the theory of irregular surfacesfundamental theorem in the theory of irregular surfaces" , or the "theorem of completeness of characteristic seriestheorem of completeness of characteristic series" ), as well as subsequent proofs by Severi, contain lacunae. The first rigorous transcendental proof of this theorem was given by Poincaré in 1910, and the algebraic proof came 50 years later (A. Grothendieck, see [5]). If the characteristic of the ground field is positive, this theorem is not valid in the general case (J. Igusa, 1955). It has been shown that, in the general case, 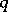 is not less than the dimension of the Picard variety.
is not less than the dimension of the Picard variety.
The non-identity of algebraic and linear equivalence on an algebraic surface implies that everywhere-regular one-dimensional differentials exist on it. The dimension of the space of such differentials in the case where 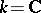 coincides with the irregularity (Castelnuovo, Severi, 1905). This fact is a special case of the equality
coincides with the irregularity (Castelnuovo, Severi, 1905). This fact is a special case of the equality 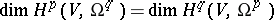 , which is valid for an arbitrary compact Kähler manifold
, which is valid for an arbitrary compact Kähler manifold 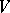 . If
. If  , this result is invalid.
, this result is invalid.
The group of divisor classes with respect to algebraic equivalence is finitely generated (see Néron–Severi group). Its rank is denoted by 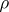 and is called the Picard number of the algebraic surface
and is called the Picard number of the algebraic surface 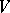 . The order of the torsion subgroup of this group is denoted by
. The order of the torsion subgroup of this group is denoted by 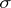 and is known as the Severi divisor of
and is known as the Severi divisor of 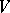 [9].
[9].
Severi also laid the foundations of the theory of zero-dimensional cycles on an algebraic surface; this theory contains many interesting problems that have not yet been solved.
e) Topological properties of an algebraic surface. A non-singular projective algebraic surface over the field of complex numbers is a compact four-dimensional oriented real manifold; in particular, the integral homology groups  and the integral cohomology groups
and the integral cohomology groups  , satisfying Poincaré duality, have been defined for such an algebraic surface. Each divisor on an algebraic surface
, satisfying Poincaré duality, have been defined for such an algebraic surface. Each divisor on an algebraic surface 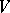 specifies a two-dimensional cycle, the divisors which are algebraically equivalent to zero correspond to cycles homologous to zero. The intersection index of divisors is identical with the topological intersection index of cycles. The following equalities are valid:
specifies a two-dimensional cycle, the divisors which are algebraically equivalent to zero correspond to cycles homologous to zero. The intersection index of divisors is identical with the topological intersection index of cycles. The following equalities are valid: 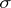 is equal to the order of the torsion group of the group
is equal to the order of the torsion group of the group  and
and  , where
, where 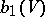 is the first Betti number of the surface
is the first Betti number of the surface  [4], [9].
[4], [9].
A method, based on the study of a linear pencil of curves on a surface and on the study of the topology of the curves which vary in this pencil, was developed for the investigation of the topology of algebraic surfaces. It was proved in this way that non-singular hyperplanes in 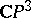 are simply connected [6]. This method was subsequently applied to studies on the topology of manifolds of higher dimension.
are simply connected [6]. This method was subsequently applied to studies on the topology of manifolds of higher dimension.
The study of étale cohomology of schemes makes it possible to formulate and prove algebraic analogues of many classical statements in the topology of algebraic surfaces for surfaces defined over a field of arbitrary characteristic. In particular, the 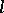 -adic cohomology groups
-adic cohomology groups 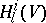 , the fundamental group and the homotopy type of an algebraic surface have all been defined [9].
, the fundamental group and the homotopy type of an algebraic surface have all been defined [9].
E. Picard [6] initiated the theory of integrals on an algebraic surface. His results were subsequently generalized to manifolds of higher dimension (W.V.D. Hodge, ca. 1940). These results yielded, in particular, the inequality 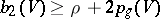 . It was proved, moreover, that the number of positive squares in the quadratic form which is defined on
. It was proved, moreover, that the number of positive squares in the quadratic form which is defined on 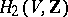 by the intersection index equals
by the intersection index equals  . The analogues of these results for fields of finite characteristic are not true. The development of the transcendental theory of algebraic surfaces is due to the work of P.A. Griffiths.
. The analogues of these results for fields of finite characteristic are not true. The development of the transcendental theory of algebraic surfaces is due to the work of P.A. Griffiths.
f) Projective immersions of an algebraic surface. In the context of the abstract concept of an algebraic variety there arose the problem of the existence of (abstract) algebraic surfaces that are not immersible in a projective space. It was proved that a non-singular complete algebraic surface is always projective [8]. There exist complete singular non-projective algebraic surfaces. There are various numerical criteria for the projectivity of an algebraic surface (cf. Ample sheaf). Any non-singular two-dimensional algebraic space is an algebraic surface.
Minimal models of algebraic surfaces.
A non-singular algebraic surface 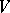 is called a minimal model if each birational morphism
is called a minimal model if each birational morphism 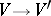 onto a non-singular algebraic surface
onto a non-singular algebraic surface 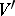 is an isomorphism. A minimal model exists in each class of birational equivalence. Except for classes of ruled surfaces, this minimal model is unique up to an isomorphism [1], [2], [8]. For an algebraic surface to constitute a minimal model it is necessary and sufficient for it not to contain exceptional curves of the first kind, i.e. irreducible curves which do contract to a non-singular point for some birational morphism. Such curves were first studied by M. Noether in 1895. They are characterized by the following requirements:
is an isomorphism. A minimal model exists in each class of birational equivalence. Except for classes of ruled surfaces, this minimal model is unique up to an isomorphism [1], [2], [8]. For an algebraic surface to constitute a minimal model it is necessary and sufficient for it not to contain exceptional curves of the first kind, i.e. irreducible curves which do contract to a non-singular point for some birational morphism. Such curves were first studied by M. Noether in 1895. They are characterized by the following requirements: 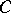 must be a rational non-singular curve and
must be a rational non-singular curve and 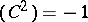 ([1], [2]). Minimal models of ruled surfaces have been completely classified.
([1], [2]). Minimal models of ruled surfaces have been completely classified.
Classification of algebraic surfaces.
According to the classification of Enriques [2], each non-singular algebraic surface over a field of characteristic zero belongs to one of the following types, up to a birational equivalence: a) ruled surfaces (cf. Ruled surface); b) two-dimensional Abelian varieties (cf. Abelian variety); c)  -surfaces (cf.
-surfaces (cf.  -surface); d) elliptic surfaces (cf. Elliptic surface); or e) general-type algebraic surfaces (cf. General-type algebraic surface). This classification is highly analogous to the classification of algebraic curves. Moreover, rational curves correspond to ruled surface, surfaces of the types b)–d) correspond to elliptic curves, and surfaces of general type correspond to curves of genus
-surface); d) elliptic surfaces (cf. Elliptic surface); or e) general-type algebraic surfaces (cf. General-type algebraic surface). This classification is highly analogous to the classification of algebraic curves. Moreover, rational curves correspond to ruled surface, surfaces of the types b)–d) correspond to elliptic curves, and surfaces of general type correspond to curves of genus 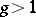 . The type of the minimal model of an algebraic surface is determined by the values of its numerical invariants. Ruled surfaces are characterized by the condition
. The type of the minimal model of an algebraic surface is determined by the values of its numerical invariants. Ruled surfaces are characterized by the condition 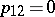 ; type-b) algebraic surfaces are characterized by the conditions
; type-b) algebraic surfaces are characterized by the conditions 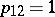 ,
, 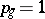 and
and 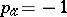 ; type-c) algebraic surfaces are characterized by the conditions
; type-c) algebraic surfaces are characterized by the conditions  and
and 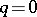 ; type-d) algebraic surfaces are characterized by the conditions
; type-d) algebraic surfaces are characterized by the conditions 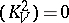 and
and  or
or  and
and 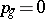 . Finally, algebraic surfaces of general type are characterized by the conditions
. Finally, algebraic surfaces of general type are characterized by the conditions 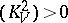 and
and  .
.
The class of ruled surfaces comprises rational surfaces (cf. Rational surface), which are characterized by the conditions 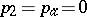 (this makes it possible to give a definite answer to the Lüroth problem in the two-dimensional case).
(this makes it possible to give a definite answer to the Lüroth problem in the two-dimensional case).
Many different results in the theory of the classification of algebraic surfaces concern the problem of the construction of an algebraic surface with given numerical invariants. Algebraic surfaces are most frequently constructed by representing them as a double covering of the projective plane with a specially selected branch curve (cf. Double plane). The values that can be assumed by the invariants 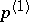 and
and 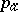 of a general-type surface are not known (1986). Some of the results obtained in connection with the classification have recently been extended to include fields of arbitrary characteristic. K. Kodaira generalized the classification results obtained by Enriques to include complex-analytic surfaces (cf. Analytic surface (in algebraic geometry)).
of a general-type surface are not known (1986). Some of the results obtained in connection with the classification have recently been extended to include fields of arbitrary characteristic. K. Kodaira generalized the classification results obtained by Enriques to include complex-analytic surfaces (cf. Analytic surface (in algebraic geometry)).
The moduli problem for algebraic surfaces.
This is the problem of the classification of algebraic surfaces up to an isomorphism. The first formula for the number of parameters (moduli) which determine an algebraic surface with given invariants 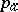 ,
, 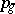 and
and 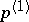 for one class of algebraic surfaces was given by Noether in 1888. Non-ruled surfaces with given invariants
for one class of algebraic surfaces was given by Noether in 1888. Non-ruled surfaces with given invariants  ,
, 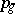 and
and 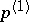 depend on
depend on  moduli, where
moduli, where
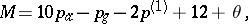 | (*) |
where 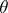 is a birational invariant of the algebraic surface under consideration [2], [9].
is a birational invariant of the algebraic surface under consideration [2], [9].
The modern theory of deformations gives the following interpretation of this classical result. The number 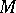 of moduli is identical with the dimension of the Zariski space tangent to the local (or, in the algebraic case, the formal) moduli scheme
of moduli is identical with the dimension of the Zariski space tangent to the local (or, in the algebraic case, the formal) moduli scheme 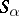 for the polarized surface
for the polarized surface 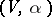 of this class of algebraic surfaces. Moreover, if
of this class of algebraic surfaces. Moreover, if
 |
is the canonical extension of the tangent sheaf 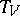 to the surface
to the surface 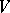 by the structure sheaf
by the structure sheaf 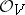 , defined by the fundamental class of the polarization
, defined by the fundamental class of the polarization 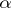 , then
, then
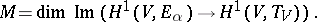 |
Formula (*) is a consequence of this exact sequence and the Riemann–Roch theorem. The number 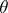 turns out to be the sum
turns out to be the sum
 |
Also, the inequality
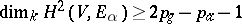 |
is valid [9]. The local moduli scheme 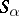 can be singular, even if
can be singular, even if 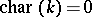 . This shows that the "actual" number of moduli, i.e.
. This shows that the "actual" number of moduli, i.e. 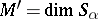 , can be less than
, can be less than 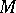 . The difference
. The difference 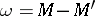 is called the number of obstructions to the deformation; the estimate
is called the number of obstructions to the deformation; the estimate 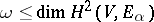 is known. The existence of a global moduli variety of an algebraic surface has been proved only for certain cases. The moduli variety for general-type surfaces and
is known. The existence of a global moduli variety of an algebraic surface has been proved only for certain cases. The moduli variety for general-type surfaces and  -surfaces exists as an analytic space or as an algebraic space.
-surfaces exists as an analytic space or as an algebraic space.
Automorphisms of algebraic surfaces.
The automorphism group 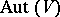 of a complete algebraic surface
of a complete algebraic surface 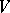 is the group of
is the group of 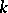 -points of a certain group scheme, the connected component
-points of a certain group scheme, the connected component 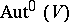 of which is an algebraic group. If
of which is an algebraic group. If 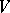 is not a ruled surface and if
is not a ruled surface and if 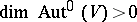 , then
, then 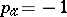 . If
. If  or if
or if 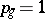 and
and 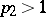 , then
, then 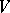 is an elliptic surface and
is an elliptic surface and  is a one-dimensional Abelian variety. In the other cases
is a one-dimensional Abelian variety. In the other cases 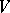 and
and  are Abelian surfaces [2]. For general-type surfaces
are Abelian surfaces [2]. For general-type surfaces  is a finite subgroup of a projective group. The group
is a finite subgroup of a projective group. The group  for
for 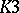 -surfaces over the field of complex numbers and for ruled surfaces has been thoroughly studied. If the surface
-surfaces over the field of complex numbers and for ruled surfaces has been thoroughly studied. If the surface 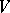 is not ruled, then
is not ruled, then 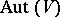 coincides with the group of birational transformations of
coincides with the group of birational transformations of 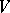 . This group has no algebraic structure for ruled surfaces and has not been thoroughly studied (cf. Cremona group). The automorphism group of affine algebraic surfaces (cf. Algebraic variety, automorphism of an) is now under intensive study.
. This group has no algebraic structure for ruled surfaces and has not been thoroughly studied (cf. Cremona group). The automorphism group of affine algebraic surfaces (cf. Algebraic variety, automorphism of an) is now under intensive study.
Algebraic surfaces over algebraically non-closed fields.
Number-theoretic problems in the theory of algebraic surfaces involve Diophantine problems (cf. Diophantine geometry). The classification of rational surfaces over non-closed fields, begun by Enriques, Comessatti and Segre, has now been completed. The group of birational automorphisms has been studied for a certain class of such surfaces.
The results of the theory of algebraic surfaces are used in the study of algebraic curves over function fields (cf. Mordell conjecture). The generalization of certain results of the theory of algebraic surfaces (minimal models, intersection theory) to the wider class of regular two-dimensional schemes [7] permits the use of the geometrical language in the study of algebraic curves over number fields.
References
| [1] | "Algebraic surfaces" Trudy Mat. Inst. Steklov. , 75 (1965) (In Russian) |
| [2] | F. Enriques, "Le superficie algebraiche" , Bologna (1949) |
| [3] | H.W.E. Jung, "Algebraische Flächen" , Hannover (1925) |
| [4] | S. Lefschetz, "L'analysis situs et la géométrie algébrique" , Gauthier-Villars (1924) |
| [5] | D. Mumford, "Lectures on curves on an algebraic surface" , Princeton Univ. Press (1966) |
| [6] | E. Picard, G. Simart, "Théorie des fonctions algébriques de deux variables indépendantes" , 1–2 , Chelsea, reprint (1971) |
| [7] | I.R. Shafarevich, "Lectures on minimal models and birational transformations of two-dimensional schemes" , Tata Inst. (1966) |
| [8] | O. Zariski, "Introduction to the problem of minimal models in the theory of algebraic surfaces" , Math. Soc. Japan (1958) |
| [9] | O. Zariski, "Algebraic surfaces" , Springer (1971) |
| [10] | O. Zariski, "An introduction to the theory of algebraic surfaces" , Springer (1969) |
Comments
One of the more important achievements is the proof of the Miyaoka–Yao–Bogomolov inequality 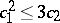 , where
, where 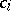 denotes the
denotes the  -th Chern class of an algebraic surface [a1]. A recent reference is [a2].
-th Chern class of an algebraic surface [a1]. A recent reference is [a2].
References
| [a1] | Y. Miyaoka, "On the Chern numbers of surfaces of general type" Invent. Math. , 42 (1977) pp. 225–237 |
| [a2] | A. van de Ven, "Compact complex surfaces" , Springer (1984) |
| [a3] | A. Beauville, "Surfaces algébrique complexes" Astérique , 54 (1958) (Translated into English as a London Math. Soc. Lecture Notes) |
| [a4] | P.A. Griffiths, J.E. Harris, "Principles of algebraic geometry" , 1–2 , Wiley (Interscience) (1978) |
Algebraic surface. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Algebraic_surface&oldid=16997