Adjoint group
of a group 
The linear group 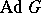 that is the image of the Lie group or algebraic group
that is the image of the Lie group or algebraic group 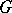 under the adjoint representation (cf. Adjoint representation of a Lie group). The adjoint group
under the adjoint representation (cf. Adjoint representation of a Lie group). The adjoint group 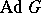 is contained in the group
is contained in the group 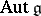 of automorphisms of the Lie algebra
of automorphisms of the Lie algebra  of
of  , and its Lie algebra coincides with the adjoint algebra
, and its Lie algebra coincides with the adjoint algebra 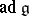 of
of 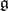 . A connected semi-simple group is a group of adjoint type (i.e. is isomorphic to its adjoint group) if and only if its roots generate the lattice of rational characters of the maximal torus; the centre of such a group is trivial. If the ground field has characteristic zero and
. A connected semi-simple group is a group of adjoint type (i.e. is isomorphic to its adjoint group) if and only if its roots generate the lattice of rational characters of the maximal torus; the centre of such a group is trivial. If the ground field has characteristic zero and 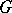 is connected, then
is connected, then 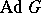 is uniquely determined by the Lie algebra
is uniquely determined by the Lie algebra  and is either called the adjoint group or the group of inner automorphisms of
and is either called the adjoint group or the group of inner automorphisms of 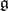 . In particular, if
. In particular, if 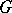 is semi-simple,
is semi-simple, 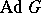 coincides with the connected component of the identity in
coincides with the connected component of the identity in 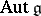 .
.
References
| [1] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) |
| [2] | J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French) |
| [3] | J.E. Humphreys, "Linear algebraic groups" , Springer (1975) |
Comments
References
| [a1] | N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) pp. Chapt. 2; 3 (Translated from French) |
Adjoint group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Adjoint_group&oldid=12627