Abel criterion

Abel's criterion for series of numbers. If the series

is convergent and if the numbers 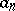form a monotone bounded sequence, then the series

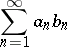is convergent.

Abel's criterion for series of functions. The series

converges uniformly on a set 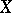if the series

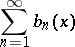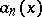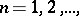converges uniformly on and if the functions , for any , form a monotone sequence that is uniformly bounded on 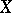. An Abel criterion for the uniform convergence of integrals

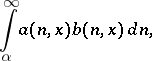which depend on a parameter 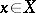, can be formulated in a similar manner.

The Abel criteria can be strengthened (see, for example, Dedekind criterion (convergence of series)). See also Dirichlet criterion (convergence of series); Abel transformation.

References

[1]	G.M. Fichtenholz, "Differential und Integralrechnung" , 1 , Deutsch. Verlag Wissenschaft. (1964)
[2]	L.D. Kudryavtsev, "Mathematical analysis" , 1 , Moscow (1973) (In Russian)
[3]	E.T. Whittaker, G.N. Watson, "A course of modern analysis" , 1–2 , Cambridge Univ. Press (1952)