Isotopy (in topology)
This term may also have other meanings, see Isotopy.
A homotopy of a topological space 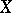 with respect to a topological space
with respect to a topological space  is a continuous family ( indexed by $t$,
is a continuous family ( indexed by $t$, 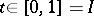 , here and throughout )
, here and throughout ) 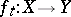 of homeomorphisms from
of homeomorphisms from  onto subsets of
onto subsets of 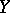 . Analogously, an isotopy is a fibrewise-continuous mapping
. Analogously, an isotopy is a fibrewise-continuous mapping  such that
such that  takes the fibre
takes the fibre 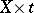 homeomorphically onto a subset of the fibre
homeomorphically onto a subset of the fibre 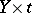 . An isotopy
. An isotopy 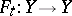 for which
for which 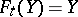 for each
for each 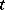 is called an isotopy of the space
is called an isotopy of the space 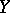 .
.
By a covering (or enveloping) isotopy for an isotopy 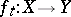 one understands an isotopy
one understands an isotopy 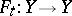 such that
such that  . Two imbeddings
. Two imbeddings 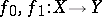 of
of 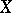 into
into 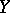 are said to be isotopic if there exist a covering isotopy
are said to be isotopic if there exist a covering isotopy 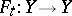 for which
for which 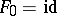 ,
, 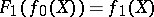 . Two spaces
. Two spaces 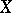 ,
, 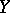 are said to be isotopy equivalent if there exist imbeddings
are said to be isotopy equivalent if there exist imbeddings 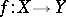 and
and 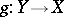 such that the composites
such that the composites 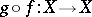 and
and 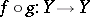 are isotopic to the identity mapping. Isotopy-equivalent spaces are also called spaces of the same isotopy type (by analogy with the homotopy type). If two spaces are homeomorphic, then they are isotopy equivalent; however, there are non-homeomorphic spaces of the same isotopy type, for example, an
are isotopic to the identity mapping. Isotopy-equivalent spaces are also called spaces of the same isotopy type (by analogy with the homotopy type). If two spaces are homeomorphic, then they are isotopy equivalent; however, there are non-homeomorphic spaces of the same isotopy type, for example, an  -dimensional ball and the same ball with a line segment glued to it (at its ends). Any homotopy invariant is an isotopy invariant, but there exist isotopy invariants, for example dimension, that are not homotopy invariants.
-dimensional ball and the same ball with a line segment glued to it (at its ends). Any homotopy invariant is an isotopy invariant, but there exist isotopy invariants, for example dimension, that are not homotopy invariants.
The fundamental problem in isotopy theory is the isotopy extension problem, that is, the problem of the existence of an isotopy 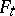 covering a given isotopy
covering a given isotopy 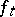 . This problem, like the general problem of finding a complete system of isotopy invariants for imbeddings, is most often considered in the category of topological manifolds and its subcategories of piecewise-linear or differentiable manifolds.
. This problem, like the general problem of finding a complete system of isotopy invariants for imbeddings, is most often considered in the category of topological manifolds and its subcategories of piecewise-linear or differentiable manifolds.
A topological isotopy 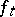 of a manifold
of a manifold 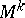 with respect to a manifold
with respect to a manifold 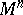 ,
,  , can be extended to a covering isotopy
, can be extended to a covering isotopy 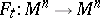 if and only if the corresponding fibrewise imbedding
if and only if the corresponding fibrewise imbedding  is locally flat (cf. Locally flat imbedding); here
is locally flat (cf. Locally flat imbedding); here 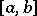 is a subinterval of
is a subinterval of 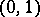 . If
. If 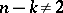 and
and 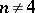 , then the isotopy
, then the isotopy  is covered by an isotopy
is covered by an isotopy  provided that the imbedding
provided that the imbedding 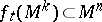 is locally flat for any
is locally flat for any  . This is not true in codimension 2 (for example, "tying a knot" on a circle in
. This is not true in codimension 2 (for example, "tying a knot" on a circle in 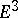 ), and therefore additional homotopy hypotheses are required for the existence of a covering isotopy. Sufficiently locally close flat imbeddings are isotopic for
), and therefore additional homotopy hypotheses are required for the existence of a covering isotopy. Sufficiently locally close flat imbeddings are isotopic for  .
.
The existence theorem for a piecewise-linear isotopy is formulated in similar fashion in the general case (under the natural condition that the corresponding fibrewise imbeddings be locally flat in the piecewise-linear sense). If 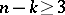 , then a piecewise-linear isotopy can always be extended, since in these codimensions a piecewise-linear imbedding is locally flat in the piecewise-linear sense. For
, then a piecewise-linear isotopy can always be extended, since in these codimensions a piecewise-linear imbedding is locally flat in the piecewise-linear sense. For 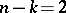 or 1 it is necessary to additionally suppose that the imbeddings
or 1 it is necessary to additionally suppose that the imbeddings 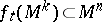 be locally flat in the piecewise-linear sense, since in these codimensions, a piecewise-linear imbedding need not to be locally flat even in the topological sense, for example, a cone over a knot.
be locally flat in the piecewise-linear sense, since in these codimensions, a piecewise-linear imbedding need not to be locally flat even in the topological sense, for example, a cone over a knot.
A differentiable isotopy can always be extended to a differentiable covering isotopy.
The problem of finding a complete system of isotopy invariants for imbeddings of a manifold 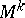 in
in 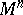 has only been solved in a few particular cases. Thus, every locally flat (in the topological sense) imbedding of spheres
has only been solved in a few particular cases. Thus, every locally flat (in the topological sense) imbedding of spheres 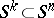 for
for 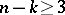 or for
or for 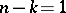 ,
, 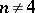 , is isotopic to the standard sphere, while if
, is isotopic to the standard sphere, while if  ,
, 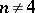 , then a locally flat sphere
, then a locally flat sphere 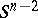 in
in 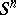 is isotopic to the standard sphere if and only if the complement
is isotopic to the standard sphere if and only if the complement 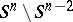 has the homotopy type of the circle (the Stallings–Brown theorem). In codimension 2 there can exist non-isotopic knots. In precisely the same way the Zeeman–Stallings theorem on the piecewise-linear isotopy (unknottedness) of piecewise-linear spheres can be stated.
has the homotopy type of the circle (the Stallings–Brown theorem). In codimension 2 there can exist non-isotopic knots. In precisely the same way the Zeeman–Stallings theorem on the piecewise-linear isotopy (unknottedness) of piecewise-linear spheres can be stated.
An invariant of piecewise-linear isotopy is finer than an invariant of topological isotopy. Thus, the problem of topological isotopy of an arbitrary homeomorphism of 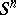 to the identity has been solved in the positive sense for
to the identity has been solved in the positive sense for 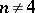 , and under the same restriction the isotopy has been proved of any two orientation-preserving homeomorphism of the sphere
, and under the same restriction the isotopy has been proved of any two orientation-preserving homeomorphism of the sphere 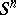 onto itself. In the piecewise-linear case, these statements can be proved by elementary methods without any restrictions. Sufficiently close homeomorphisms of a topological manifold onto itself are isotopic; on the other hand, there exist arbitrarily close, piecewise-linear non-isotopic, piecewise-linear homeomorphisms of piecewise-linear manifolds, for example, of the
onto itself. In the piecewise-linear case, these statements can be proved by elementary methods without any restrictions. Sufficiently close homeomorphisms of a topological manifold onto itself are isotopic; on the other hand, there exist arbitrarily close, piecewise-linear non-isotopic, piecewise-linear homeomorphisms of piecewise-linear manifolds, for example, of the  -dimensional tori for
-dimensional tori for 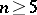 .
.
In contrast with the topological and piecewise-linear cases, it is by no means true that any two diffeomorphisms of an  -sphere onto itself are differentiably isotopic. The isotopy classes of differentiable imbeddings of a sphere
-sphere onto itself are differentiably isotopic. The isotopy classes of differentiable imbeddings of a sphere 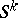 into
into  have been studied in detail for any
have been studied in detail for any 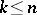 . If
. If 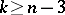 , then there exist a topological space
, then there exist a topological space 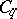 ,
, 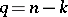 , such that the homotopy groups
, such that the homotopy groups 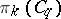 are in one-to-one correspondence with the imbedding classes of
are in one-to-one correspondence with the imbedding classes of  into
into 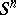 . Here
. Here 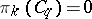 for
for 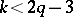 , while
, while 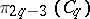 is
is 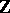 or
or 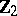 depending on
depending on 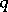 being even or odd. Thus, the standard
being even or odd. Thus, the standard 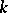 -sphere
-sphere 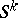 , imbedded in
, imbedded in 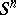 , can for
, can for 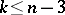 be knotted in the differentiable sense, that is, there exist imbeddings
be knotted in the differentiable sense, that is, there exist imbeddings  that are not differentiably isotopic to the standard imbedding. These knots are called Haefliger knots. If
that are not differentiably isotopic to the standard imbedding. These knots are called Haefliger knots. If 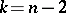 , then the differentiable knots
, then the differentiable knots  can be knotted in the topological sense; however, there are considerably more of these than of the topological or piecewise-linear ones; for odd
can be knotted in the topological sense; however, there are considerably more of these than of the topological or piecewise-linear ones; for odd  they have been completely classified. If
they have been completely classified. If 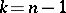 ,
, 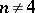 , then any differentiable imbedding
, then any differentiable imbedding 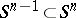 is differentiably isotopic to the standard imbedding.
is differentiably isotopic to the standard imbedding.
The fact that there exist diffeomorphisms of 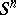 onto itself that are not isotopic to the identity leads to the existence of non-trivial differentiable structures on spheres of dimension
onto itself that are not isotopic to the identity leads to the existence of non-trivial differentiable structures on spheres of dimension 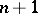 . Although every homeomorphism of
. Although every homeomorphism of 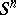 ,
, 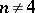 , onto itself can be approximated by a diffeomorphism, not all close diffeomorphisms of a sphere are differentiably isotopic, that is, a diffeomorphism of a sphere onto itself can by an infinitesimal perturbation be transformed in one not isotopic to it.
, onto itself can be approximated by a diffeomorphism, not all close diffeomorphisms of a sphere are differentiably isotopic, that is, a diffeomorphism of a sphere onto itself can by an infinitesimal perturbation be transformed in one not isotopic to it.
References
| [1] | L.V. Keldysh, "Topological imbeddings in Euclidean space" Proc. Steklov Inst. Math. , 81 (1968) Trudy Mat. Inst. Steklov. , 81 |
| [2] | C.P. Rourke, B.J. Sanderson, "Introduction to piecewise-linear topology" , Springer (1972) |
| [3] | S.P. Novikov, "Differentiable sphere bundles" Izv. Akad. Nauk SSSR Ser. Mat. , 29 : 1 (1965) pp. 71–96 (In Russian) |
| [4] | A.V. Chernavskii, "Local contractibility of the group of homeomorphisms of a manifold" Math. USSR Sb. , 8 : 3 (1969) pp. 287–333 |
| [5] | T. Rushing, "Topological embeddings" , Acad. Press (1973) |
| [6] | L.C. Siebenmann, R.C. Kirby, "Foundational essays on topological manifolds, smoothings, and triangulations" , Princeton Univ. Press (1977) |
Comments
Isotopies are also very important in infinite-dimensional topology (cf. Infinite-dimensional space). For example, if  and
and 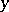 are points in the Hilbert cube
are points in the Hilbert cube 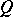 , then there exists an isotopy
, then there exists an isotopy 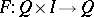 such that
such that 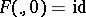 and
and 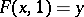 .
.
References
| [a1] | J. van Mill, "Infinite-dimensional topology, prerequisites and introduction" , North-Holland (1988) |
Isotopy (in topology). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Isotopy_(in_topology)&oldid=50982