Singular measures

Two (positive) measures 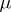and , defined on a locally compact space 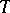, such that .

Two measures 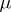and are mutually singular if and only if there exist in 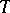two disjoint sets 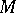and 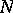such that 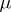is concentrated on and on .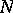

References

[1]	N. Bourbaki, "Elements of mathematics. Integration" , Addison-Wesley (1975) pp. Chapt.6;7;8 (Translated from French)

Comments

The second characterization in the main article above holds if and are -additive 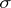-finite measures on an abstract measurable space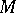, and and belong to the -field.

Mutually-singular measures are also called singular measures or orthogonal measures.

Instead of "concentrated on" one also uses "supported in" (cf. also Support of a measure).

References

[a1]	P.R. Halmos, "Measure theory" , v. Nostrand (1950)
[a2]	E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965)