Urn model
One of the simplest models in probability theory. A description of an urn model is as follows: Consider some vessel — an urn — with black and white balls. One ball is drawn at random from the urn, and then it is returned to the urn together with  balls of the same colour as the ball drawn and
balls of the same colour as the ball drawn and 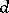 balls of the other colour. After mixing the balls in the urn, the procedure is repeated a certain number of times. It is assumed that initially the urn contains
balls of the other colour. After mixing the balls in the urn, the procedure is repeated a certain number of times. It is assumed that initially the urn contains  white and
white and  black balls. The numbers
black balls. The numbers  and
and 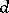 , the parameters of the urn model, may also be negative.
, the parameters of the urn model, may also be negative.
The urn model offers a convenient means for calculating certain basic probabilities by use of conditional probabilities. For different values of the parameters  and
and 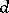 many known models of probability theory are obtained: for
many known models of probability theory are obtained: for 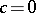 ,
, 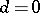 the model of random choice with replacement (cf. Bernoulli trials), for
the model of random choice with replacement (cf. Bernoulli trials), for 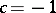 ,
, 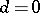 the model of random choice without replacement, for
the model of random choice without replacement, for 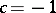 ,
, 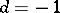 the Ehrenfest diffusion model, for
the Ehrenfest diffusion model, for 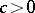 ,
,  the Pólya urn model, etc. These special cases serve as models for many real phenomena and provide methods for studying them. For example, the Pólya urn model is used for the description of epidemics, in which the occurrence of any event increases the likelihood of a subsequent occurrence of this event. Within the framework of urn models many distributions in probability theory can be introduced, such as the binomial, the hypergeometric, the geometric, and the Pólya distributions. The negative binomial distribution and the Poisson distribution arise as limit distributions from certain urn models.
the Pólya urn model, etc. These special cases serve as models for many real phenomena and provide methods for studying them. For example, the Pólya urn model is used for the description of epidemics, in which the occurrence of any event increases the likelihood of a subsequent occurrence of this event. Within the framework of urn models many distributions in probability theory can be introduced, such as the binomial, the hypergeometric, the geometric, and the Pólya distributions. The negative binomial distribution and the Poisson distribution arise as limit distributions from certain urn models.
References
| [1] | W. Feller, "An introduction to probability theory and its applications", 1–2, Wiley (1957–1971) |
Comments
References
| [a1] | N.L. Johnson, S. Kotz, "Urn models and their application" , Wiley (1977) |
Urn model. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Urn_model&oldid=25971