Removable set

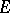of points of the complex plane 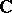for a certain class 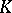of functions analytic in a domain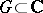

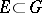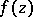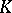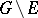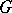A compact set such that any function of class in can be continued as a function of class to the whole domain . The situation may be described in other words by saying that "the set E is set, removable for a class of functionsremovable for the class K" or that "E is a null-set for the class K" , briefly: 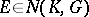. It is assumed that the complement 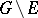is a domain and that the class 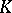is defined for any domain.

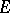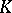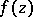According to another definition, a set is removable for a class , , if the fact that is a function of class 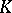in the complement 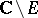implies that . Here the membership relations and 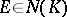are generally speaking not equivalent.

A first result on removable sets was the classical Cauchy–Riemann theorem on removable singularities: If a function 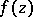is analytic and bounded in a punctured neighbourhood of a point 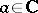, then it can be continued analytically to . A wider statement of the question (Painlevé's problem) is due to P. Painlevé: To find necessary and sufficient conditions on a set 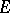in order that 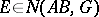, where 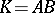is the class of all bounded analytic functions (cf. [1]). Painlevé himself found a sufficient condition: 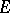must have linear Hausdorff measure zero. Necessary and sufficient conditions for Painlevé's problem were obtained by L.V. Ahlfors (cf. [2]): if and only if 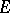has zero analytic capacity. There exists an example of a set 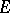of positive length but zero analytic capacity. On removable sets for different classes of analytic functions of one complex variable and related unsolved problems see [3], [4], [6], [9].

In the case of analytic functions 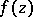of several complex variables 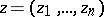, , 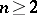the statement of the problem on removable sets is changed by virtue of the classical Osgood–Brown theorem: If 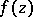is a regular analytic function in a domain 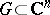, except possibly on a compact set 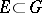for which the complement 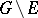is connected, then 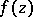can be continued analytically to the whole domain 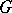. For other theorems on removable sets for 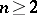, as well as connections with the concept of a domain of holomorphy, see e.g. [7], [10].

The problem of removable sets can also be posed for harmonic, subharmonic and other functions. E.g., let 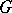be a domain in Euclidean space 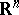, , 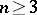let 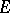be compact, , let 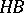be the class of bounded harmonic functions, and let 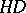be the class of harmonic functions with finite Dirichlet integral. Then the membership relations 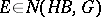and 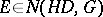are equivalent and are valid if and only if the capacity of 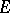is zero (cf. [5], [8]).

References

[1]	L. Zoretti, "Leçons sur la prolongement analytique" , Gauthier-Villars (1911)
[2]	L.V. Ahlfors, "Bounded analytic functions" Duke Math. J. , 14 : 1 (1947) pp. 1–11 MR0021108 Zbl 0030.03001
[3]	K. Nohiro, "Cluster sets" , Springer (1960)
[4]	S.Ya. Khavinson, "Analytic functions of bounded type" Itogi Nauk. Mat. Anal. 1963 (1965) pp. 5–80 (In Russian)
[5]	L. Carleson, "Selected problems on exceptional sets" , v. Nostrand (1967) MR0225986 Zbl 0189.10903
[6]	M.S. Mel'nikov, S.O. Sinanyan, "Aspects of approximation theory for functions of one complex variable" J. Soviet Math. , 5 : 5 (1976) pp. 688–752 Itogi Nauk. i Tekhn. Sovremen. Probl. Mat. , 4 (1975) pp. 143–250 Zbl 0375.30014
[7]	B.V. Shabat, "Introduction of complex analysis" , 2 , Moscow (1985) (In Russian) Zbl 0578.32001 Zbl 0574.30001
[8]	W.K. Hayman, P.B. Kennedy, "Subharmonic functions" , 1 , Acad. Press (1976) MR0460672 MR0419791 MR0412442 MR0442324 Zbl 0419.31001 Zbl 0339.31003 Zbl 0328.33011
[9]	E.P. Dolzhenko, "Elimination of singularities of analytic functions" Uspekhi Mat. Nauk , 18 : 4 (1963) pp. 135–142 (In Russian)
[10]	L.J. Riihentaus, "Removable singularities of analytic functions of several complex variables" Math. Z. , 158 (1978) pp. 45–54 MR0484286 Zbl 0351.32014

Comments

The Osgood–Brown theorem is also called Hartogs' theorem, cf. Hartogs theorem.

See also Analytic continuation; Analytic set; Removable singular point.

For quite general continuation results see [a2]; Riemann's theorem has an analogue in : Bounded analytic functions extend analytically across subvarieties of codimension 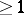, while all analytic functions can be analytically continued across subvarieties of codimension 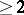. See [a1].

References

[a1]	R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) pp. Chapt. 1, Section G MR0180696 Zbl 0141.08601
[a2]	R. Harvey, J. Polking, "Removable singularities of solutions of linear partial differential equations" Acta Math. , 125 (1970) pp. 39–55 MR0279461 Zbl 0214.10001
[a3]	J.B. Garnett, "Analytic capacity and measure" , Lect. notes in math. , 297 , Springer (1972) MR0454006 Zbl 0253.30014
[a4]	E.M. Chirka, "Complex analytic sets" , Kluwer (1989) (Translated from Russian) MR1111477 Zbl 0683.32002