Differential equation, partial, discontinuous initial (boundary) conditions

A problem involving partial differential equations in which the functions specifying the initial (boundary) conditions are not continuous.

For instance, consider the second-order hyperbolic equation

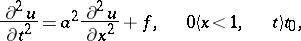and pose for it the mixed problem with initial conditions

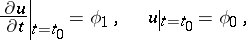and boundary conditions

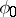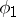In this case the discontinuities of the initial functions and entail discontinuities of and 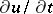along the characteristic rays and 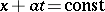, and the measure of discontinuity

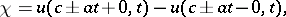or

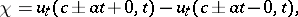where 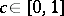is a discontinuity point of the function 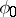or 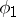, satisfies the equation

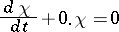along the characteristic ray, i.e. 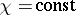. Similar results are valid for second-order hyperbolic equations with variable coefficients:

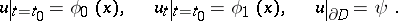In this case the discontinuities of the initial functions and the boundary conditions also entail discontinuities in and along characteristic rays, which can be determined from the systems of equations

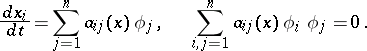The measure of discontinuity 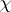satisfies the equation:

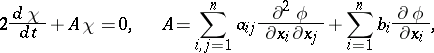where the function 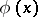defines the characteristic surface in the form of the equation .

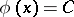In the case of equations of elliptic type the discontinuities of the boundary conditions do not propagate inside 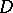because in this case the characteristic rays are complex. For equations of elliptic type studies were made of the existence and uniqueness of the solution, and of the solution satisfying the boundary conditions. Thus, it has been proved for second-order elliptic equations in an arbitrary domain,

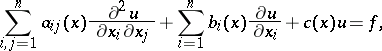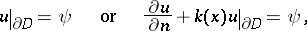that if the boundary function 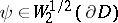for the first boundary condition and 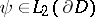for the second boundary condition, then there exists a generalized solution in 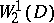which satisfies the boundary condition on the average, i.e. 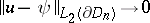, where the surfaces 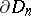approximate the surface 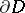. In the case of parabolic (and also elliptic) equations, the discontinuities do not propagate inside 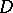if discontinuities are present in the initial or in the boundary conditions. Problems of the existence and uniqueness of a generalized solution to the boundary condition have also been studied for these problems.

References

[1]	A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian) MR104888
[2]	V.S. Vladimirov, "Equations of mathematical physics" , MIR (1984) (Translated from Russian) MR0764399 Zbl 0954.35001 Zbl 0652.35002 Zbl 0695.35001 Zbl 0699.35005 Zbl 0607.35001 Zbl 0506.35001 Zbl 0223.35002 Zbl 0231.35002 Zbl 0207.09101
[3]	A.V. Bitsadze, "Equations of mathematical physics" , MIR (1980) (Translated from Russian) MR0587310 MR0581247 Zbl 0499.35002
[4]	R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) MR0195654
[5]	L. Bers, F. John, M. Schechter, "Partial differential equations" , Interscience (1964) MR0163043 Zbl 0126.00207
[6]	O.A. Ladyzhenskaya, N.N. Ural'tseva, "Linear and quasilinear elliptic equations" , Acad. Press (1968) (Translated from Russian) MR0244627 Zbl 0177.37404 Zbl 0164.13002
[7]	O.A. Ladyzhenskaya, V.A. Solonnikov, N.N. Ural'tseva, "Linear and quasilinear parabolic equations" , Amer. Math. Soc. (1968) (Translated from Russian) Zbl 0182.43204 Zbl 0179.15003 Zbl 0164.12302 Zbl 0142.37603 Zbl 0149.31301 Zbl 0113.30701
[8]	A. Friedman, "Partial differential equations of parabolic type" , Prentice-Hall (1964) MR0181836 Zbl 0144.34903
[9]	C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian) MR0284700 Zbl 0198.14101
[10]	S. Agmon, A. Douglis, L. Nirenberg, "Estimates near the boundary for solutions of elliptic equations" Comm. Pure Appl. Math. , 12 (1959) pp. 623–727 Zbl 0093.10401

Comments

Far-reaching results have been obtained recently concerning harmonic analysis of singularities and propagation of singularities, cf. [a1].

References

[a1]	L.V. Hörmander, "The analysis of linear partial differential operators" , 3 , Springer (1985) MR1540773 MR0781537 MR0781536 Zbl 0612.35001 Zbl 0601.35001