Mapping
$ \def\P{\mathcal P} % power set \def\iff{\Longleftrightarrow} $
Mapping, or abbreviated map, is one of many synonyms used for function. In particular, the term map(ping) is used in general contexts, such as set theory, but usage is not restricted to these cases.
The mapping concept in set theory
In set theory mappings are special binary relations.
A mapping $f$ from a set $A$ to a set $B$ is an (ordered) triple $ f = (A,B,G_f) $ where $ G_f \subset A \times B $ such that
- (a) if $ (x,y) $ and $ (x,y') \in G_f $ then $ y=y' $, and
- (b) the projection $ \pi_1 (G_f) = \{ x \mid (x,y) \in G_f \} = A $.
Condition (a) expresses that $f$ is single-valued. and
condition (b) that it is defined on $A$.
$A$ is the domain, $B$ is the codomain, and $G_f$ is the graph of the mapping.
Therefore, in this setting, mappings are equal if and only if
all three corresponding components (domain, codomain, and graph) are equal.
The mapping is usually denoted as $ f : A \to B $, and $ a \mapsto f(a) $
where $ f(a) := b \iff (a,b) \in G_f $ is the value of $f$ at $a$.
Remark:
Sometimes only the graph $G_f$ is used to represent a function.
In this case two mappings are equal if they have the same graph,
and one may allow graphs that are not sets but classes.
While the domain of the function can be obtained as projection $ \pi_1 (G_f) $ of the first component,
the projection $ \pi_2 (G_f) $ of the second component does not produce the codomain but only the image of the domain.
Thus the concept of surjectivity is not applicable.
Composition
Two mappings can be composed if the codomain of one mapping is a subset of the domain of the other mapping:
For $ f=(A,B,G_f) $ and $ g=(C,D,G_g) $ with $ B \subset C $ the composition $ g \circ f $ is the mapping $ (A,D,G) $ with
- $ G := \{ (a,g(f(a))) \mid a \in A \} = \{ (a,c) \mid (\exists b \in B) ( (a,b) \in G_f \land (b,c) \in G_g ) \} $.
Remarks:
(a) The condition $ B \subset C $ can be relaxed to $ f(A) \subset C $.
(b) If only graphs are used then the graph of the composition is defined (as above) by
- $ G_{g \circ f} := \{ (a,c) \mid (\exists b ) ( (a,b) \in G_f \land (b,c) \in G_g ) \} $
but may turn out to be empty.
Induced mappings
Every mapping $ f : A \to B $ induces two mappings between the power sets $\P(A)$ and $\P(B)$.
- $ f_\ast : \P(A) \to \P(B) $ defined by $ f_\ast (S) := \{ f(a) \mid a \in S \}$ for $ S \subset A $
and
- $ f^\ast : \P(B) \to \P(A) $ defined by $ f^\ast (T) := \{ a \mid f(a) \in T \}$ for $ T \subset B $
$ f_\ast (S) $ is called the image of $S$ under $f$, usually denoted as $f(S)$, and $ f^\ast (T) $ is called the inverse image of $T$ under $f$, usually denoted as $f^{-1}(T)$, but one has to be aware that these common notations may be ambiguous in certain situations.
References
| [1] | N. Bourbaki, "Elements of mathematics. Theory of sets" , Addison-Wesley (1968) (Translated from French) |
| [2] | N. Bourbaki, "Elements of mathematics. General topology" , Addison-Wesley (1966) (Translated from French) |
| [3] | J.L. Kelley, "General topology" , Springer (1975) |
Comments
For a mapping 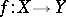 , the set
, the set 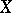 is also called the source of
is also called the source of 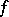 , while
, while 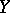 is also called the target of
is also called the target of 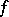 , [a3].
, [a3].
References
| [a1] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) |
| [a2] | P.R. Halmos, "Naive set theory" , v. Nostrand (1961) |
| [a3] | P.M. Cohn, "Universal algebra" , Reidel (1981) |
Mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Mapping&oldid=24212