Weil cohomology

Cohomology of algebraic varieties with coefficients in a field of characteristic zero, with formal properties required to obtain the Lefschetz formula for the number of fixed points. The necessity for such a theory was pointed out by A. Weil [1], who showed that the rationality of the zeta-function and 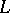-function of a variety over a finite field follow from the Lefschetz formula, whereas the remaining hypotheses about the zeta-function can naturally be formulated in cohomological terms. Let the variety 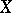be a projective smooth connected scheme over a fixed algebraically closed field 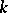and let 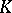be a field of characteristic zero. 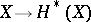Then Weil cohomology with coefficient field is a contravariant functor from the category of varieties into the category of finite-dimensional graded anti-commutative 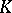-algebras, which satisfies the following conditions:

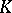1) If , then is isomorphic to , and the mapping

defined by the multiplication in , is non-degenerate for all ;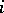

2) (Künneth formula);

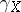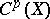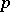3) Mapping of cycles. There exists a functorial homomorphism from the group of algebraic cycles in of codimension into 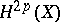which maps the direct product of cycles to the tensor product and is non-trivial in the sense that, for a point , 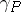becomes the canonical imbedding of into 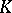. The number

is known as the 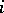-th Betti number of the variety .

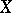Examples. If 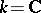, classical cohomology of complex manifolds with coefficients in 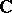is a Weil cohomology. If 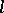is a prime number distinct from the characteristic of the field , then étale 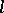-adic cohomology

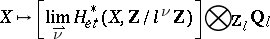is a Weil cohomology with coefficients in the field .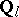

The Lefschetz formula

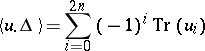is valid for Weil cohomology. In the above formula, 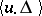is the intersection index in 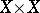of the graph 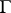of the morphism 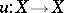with the diagonal 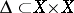, which may also be interpreted as the number of fixed points of the endomorphism , while 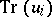is the trace of the endomorphism 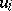which is induced by in 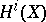. Moreover, this formula is also valid for correspondences, i.e. elements .

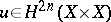References

[1]	A. Weil, "Numbers of solutions of equations in finite fields" Bull. Amer. Math. Soc. , 55 (1949) pp. 497–508 MR0029393 Zbl 0032.39402
[2]	S.L. Kleiman, "Algebraic cycles and the Weil conjectures" A. Grothendieck (ed.) J. Giraud (ed.) et al. (ed.) , Dix exposés sur la cohomologie des schémas , North-Holland & Masson (1968) pp. 359–386 MR0292838 Zbl 0198.25902

References

[a1]	A. Grothendieck, "The cohomology theory of abstract algebraic varieties" J.A. Todd (ed.) , Proc. Internat. Congress Mathematicians (Edinburgh, 1958) , Cambridge Univ. Press (1960) pp. 103–118 MR0130879 Zbl 0119.36902
[a2]	A. Grothendieck, I. Bucur, C. Honzel, L. Illusie, J.-P. Jouanolou, J.-P. Serre, "Cohomologie -adique et fonctions . SGA 5" , Lect. notes in math. , 589 , Springer (1977) MR491704
[a3]	J.S. Milne, "Etale cohomology" , Princeton Univ. Press (1980) MR0559531 Zbl 0433.14012
[a4]	E. Freitag, R. Kiehl, "Étale cohomology and the Weil conjecture" , Springer (1988) MR0926276 Zbl 0643.14012
[a5]	R. Hartshorne, "Algebraic geometry" , Springer (1977) pp. 272 MR0463157 Zbl 0367.14001