Tangent sheaf
in algebraic geometry
The sheaf  on an algebraic variety or scheme
on an algebraic variety or scheme  over a field
over a field  , whose sections over an open affine subspace
, whose sections over an open affine subspace 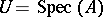 are the
are the  -modules of
-modules of  -derivations
-derivations  of the ring
of the ring  . An equivalent definition is that
. An equivalent definition is that  be the sheaf of homomorphisms
be the sheaf of homomorphisms  of the sheaf of differentials
of the sheaf of differentials 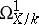 into the structure sheaf
into the structure sheaf 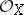 (see Derivations, module of).
(see Derivations, module of).
For any rational  -point
-point  , the stalk
, the stalk  of the sheaf
of the sheaf  is identical to the Zariski tangent space
is identical to the Zariski tangent space 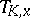 to
to 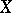 at
at  , that is, to the vector
, that is, to the vector 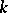 -space
-space 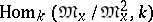 , where
, where 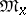 is the maximal ideal of the local ring
is the maximal ideal of the local ring 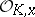 . Instead of the tangent sheaf
. Instead of the tangent sheaf  one can use the sheaf of germs of sections of the vector bundle
one can use the sheaf of germs of sections of the vector bundle  dual to
dual to  (or the tangent bundle to
(or the tangent bundle to  ). In the case when
). In the case when  is a smooth connected
is a smooth connected  -scheme,
-scheme, 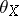 is a locally free sheaf on
is a locally free sheaf on  of rank equal to the dimension of
of rank equal to the dimension of 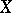 .
.
References
| [1] | I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) MR0447223 Zbl 0362.14001 |
Comments
References
| [a1] | R. Hartshorne, "Algebraic geometry" , Springer (1977) pp. Sect. IV.2 MR0463157 Zbl 0367.14001 |
Tangent sheaf. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tangent_sheaf&oldid=23990