Stratification

A decomposition of a (possibly infinite-dimensional) manifold into connected submanifolds of strictly-diminishing dimensions.

Comments

Usually a "stratification" of a space means more than just some decomposition into connected pieces with diminishing dimensions.

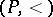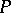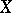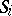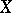Let be a partially ordered set. A -decomposition of a topological space is a locally finite collection of subspaces of , labelled by the elements of 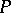, such that:

1) 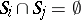if ;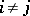

2) 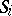is locally closed for all ;

3) ;

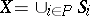4) if 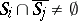, then 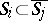(and this is equivalent to 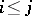in ).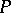

As an example, consider the subset of 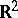given by the inequality 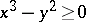divided into the four pieces , , , .

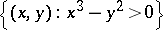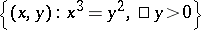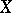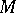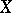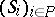Now let be a subset of a smooth manifold . A stratification of is a -decomposition for some partially ordered set 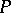such that each of the pieces is a smooth submanifold of .

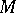The stratification is called a Whitney stratification if for every pair of strata 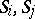with 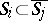the following Whitney's conditions 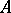and hold. Suppose that a sequence of points converges to 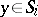and a sequence of points 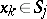also converges to . Suppose, moreover, that the tangent planes 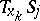converge to some limiting plane 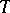and that the secant lines 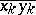converge to some line 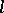(all with respect to some local coordinate system around in the ambient manifold ). Then

A) ;

B) .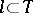

Condition B) implies in fact condition A).

A few facts and theorems concerning Whitney stratifications are as follows. Any closed subanalytic subset of an analytic manifold admits a Whitney stratification, [a5]. In particular, algebraic sets in 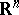, i.e. sets given by the vanishing of finitely many polynomials (cf. also Semi-algebraic set), can be Whitney stratified. Whitney stratified spaces can be triangulated, [a4].

References

[a1]	J. Mather, "Notes on topological stability" , Harvard Univ. Press (1970) (Mimeographed notes)
[a2]	C.G. Gibson, K. Wirthmüller, A.A. du Plessis, E.J.N. Looijenga, "Topological stability of smooth mappings" , Lect. notes in math. , 552 , Springer (1976) MR0436203 Zbl 0377.58006
[a3]	M. Goresky, "Stratified Morse theory" , Springer (1988) MR0932724 Zbl 0639.14012
[a4]	F. Johnson, "On the triangulation of stratified sets and singular varieties" Trans. Amer. Math. Soc. , 275 (1983) pp. 333–343 MR0678354 Zbl 0511.58007
[a5]	H. Hironaka, "Subanalytic sets" , Number theory, algebraic geometry and commutative algebra , Kinokuniya (1973) pp. 453–493 MR0377101 Zbl 0297.32008
[a6]	H. Whitney, "Tangents to an analytic variety" Ann. of Math. , 81 (1965) pp. 496–549 MR0192520 Zbl 0152.27701
[a7]	H. Whitney, "Local properties of analytic varieties" S. Cairns (ed.) , Differentiable and Combinatorial Topology , Princeton Univ. Press (1965) pp. 205–244 MR0188486 Zbl 0129.39402
[a8]	R. Thom, "Propriétés différentielles locales des ensembles analytiques" , Sem. Bourbaki , Exp. 281 (1964/5) MR1608789 Zbl 0184.31402