Congruence modulo a prime number
A congruence in which the modulus is a prime number. A distinguishing feature of the theory of congruences modulo a prime number is the fact that the residue classes modulo 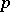 form a finite field of
form a finite field of 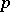 elements. Congruences modulo a prime number can therefore be treated as equations over finite prime fields and algebraic-geometric methods, as well as methods of number theory, can be used to study them.
elements. Congruences modulo a prime number can therefore be treated as equations over finite prime fields and algebraic-geometric methods, as well as methods of number theory, can be used to study them.
One of the basic questions in the theory of congruences with one variable  , which is of great significance to algebraic number theory, coding theory and other branches of mathematics, is the question of the study of the laws of decomposition
, which is of great significance to algebraic number theory, coding theory and other branches of mathematics, is the question of the study of the laws of decomposition
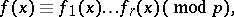 |
modulo a prime number 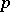 , of arbitrary integer polynomials
, of arbitrary integer polynomials 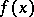 into irreducible factors.
into irreducible factors.
A second basic question in the theory of congruences modulo a prime number 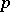 with
with  variables is the question of the number of solutions of a congruence equation
variables is the question of the number of solutions of a congruence equation
 |
when 
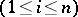 vary independently of each other over either the whole set of residue classes modulo
vary independently of each other over either the whole set of residue classes modulo 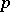 (problems of complete residue systems), or over a particular part of it (problems of incomplete residue systems).
(problems of complete residue systems), or over a particular part of it (problems of incomplete residue systems).
The first results of the research into the question of the number of solutions of quadratic and bi-quadratic congruences with two variables were obtained by C.F. Gauss [1] and J.L. Lagrange [2]. E. Artin [3] established a link between the problem of the number of solutions of the hyper-elliptic congruences 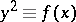 (
(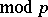 ) on a complete residue system modulo the prime number
) on a complete residue system modulo the prime number 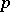 and the Riemann hypothesis for
and the Riemann hypothesis for 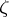 -functions of algebraic function fields with a finite field of constants, which were introduced by him. In particular, he stated the hypothesis that for the number
-functions of algebraic function fields with a finite field of constants, which were introduced by him. In particular, he stated the hypothesis that for the number 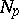 of solutions of the congruence
of solutions of the congruence 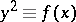 (
(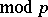 ), where the polynomial
), where the polynomial 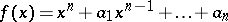 is not the square of another polynomial modulo
is not the square of another polynomial modulo 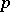 , the estimate
, the estimate
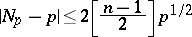 |
is correct (here 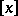 is the integer part of the number
is the integer part of the number  ).
).
Artin's hypothesis was first proved by H. Hasse [6] for the case of the elliptic congruences
 |
A. Weil [8] subsequently extended the method of Hasse to cover the general case and obtained the estimate
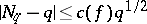 |
for the number 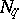 of solutions of the equation
of solutions of the equation  in elements of the field
in elements of the field  , consisting of
, consisting of 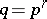 elements, where
elements, where 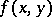 is an absolutely-irreducible polynomial with coefficients from
is an absolutely-irreducible polynomial with coefficients from 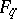 . The Hasse–Weil method is complicated and requires the use of modern abstract algebraic geometry. A simple and purely arithmetic method of proving the results of Hasse and Weil can be found in [7].
. The Hasse–Weil method is complicated and requires the use of modern abstract algebraic geometry. A simple and purely arithmetic method of proving the results of Hasse and Weil can be found in [7].
Congruences modulo a prime number with  variables are less widely studied. The following theorem can be used here as a general result. Let
variables are less widely studied. The following theorem can be used here as a general result. Let 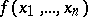 be an absolutely-irreducible polynomial with integer coefficients. Then for the number
be an absolutely-irreducible polynomial with integer coefficients. Then for the number 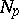 of solutions of the congruence
of solutions of the congruence
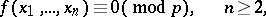 |
the estimate
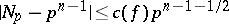 |
holds, where the constant 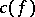 does not depend on
does not depend on 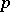 . A better estimate has been obtained by P. Deligne [9].
. A better estimate has been obtained by P. Deligne [9].
For results on congruences modulo a prime number on an incomplete residue system, see Vinogradov hypotheses; Two-term congruence; Distribution of power residues and non-residues.
References
| [1] | C.F. Gauss, "Untersuchungen über höhere Arithmetik" , Springer (1889) (Translated from Latin) MR0188045 Zbl 21.0166.04 |
| [2] | J.L. Lagrange, "Démonstration d'un théorème d'arithmétique" , Oeuvres , 3 , Paris (1869) pp. 189–201 |
| [3] | E. Artin, "Quadratische Körper in Gebiete der höheren Kongruenzen II" Math. Z. , 19 (1924) pp. 207–246 |
| [4] | I.M. Vinogradov, "Selected works" , Springer (1985) (Translated from Russian) MR0807530 Zbl 0577.01049 |
| [5] | H. Hasse, "Zahlentheorie" , Akademie Verlag (1963) MR0153659 Zbl 1038.11500 |
| [6] | H. Hasse, "Abstrakte Begründung der komplexen multiplication und Riemannsche Vermutung in Funktionenkörpern" Abh. Math. Sem. Hamburg Univ. , 10 (1934) pp. 325–347 |
| [7] | S.A. Stepanov, "A constructive method in the theory of equations over finite fields" Trudy Mat. Inst. Steklov. , 132 (1973) pp. 237–246 (In Russian) |
| [8] | A. Weil, "Courbes algébriques et variétés abéliennes. Sur les courbes algébriques et les varietés qui s'en deduisent" , Hermann (1948) |
| [9] | P. Deligne, "La conjecture de Weil I" Publ. Math. IHES , 43 (1974) pp. 273–307 MR0340258 Zbl 0314.14007 Zbl 0287.14001 |
Comments
A polynomial  over
over 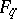 is absolutely irreducible if it is still irreducible over the algebraic closure
is absolutely irreducible if it is still irreducible over the algebraic closure 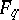 of
of 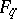 . For some material on polynomials over finite fields and their factorizations in the context of coding theory cf. [a1].
. For some material on polynomials over finite fields and their factorizations in the context of coding theory cf. [a1].
References
| [a1] | F.J. MacWilliams, N.J.A. Sloane, "The theory of error-correcting codes" , I-II , North-Holland (1977) |
| [a2] | G.H. Hardy, E.M. Wright, "An introduction to the theory of numbers" , Oxford Univ. Press (1979) pp. Chapts. 5; 7; 8 MR0568909 Zbl 0423.10001 |
Congruence modulo a prime number. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Congruence_modulo_a_prime_number&oldid=23797