Turán number
A collection 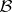 of subsets of size
of subsets of size  ( "blocks" ) of a ground set
( "blocks" ) of a ground set 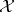 of size
of size  is said to form a Turán
is said to form a Turán  -system if each
-system if each 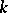 -element subset of
-element subset of 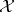 contains at least one block. The Turán number
contains at least one block. The Turán number 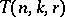 is the minimum size of such a collection. P. Turán introduced these numbers in [a6]. The related dual notion is that of the covering number
is the minimum size of such a collection. P. Turán introduced these numbers in [a6]. The related dual notion is that of the covering number 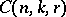 , defined to be the smallest number of blocks needed to cover (by inclusion) each
, defined to be the smallest number of blocks needed to cover (by inclusion) each 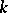 -element subset. Several recursions are known; e.g. in [a2] it is shown that
-element subset. Several recursions are known; e.g. in [a2] it is shown that
 |
Also, the limit
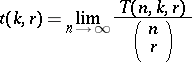 |
is known to exist, though the values of 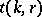 are known only for
are known only for 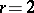 . These facts and the ones that follow are based on an extensive survey by A. Sidorenko ([a5]):
. These facts and the ones that follow are based on an extensive survey by A. Sidorenko ([a5]):
i) 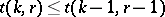 .
.
ii)  [a1].
[a1].
iii) It has been conjectured that 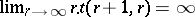 [a1].
[a1].
iv) 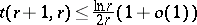 [a4].
[a4].
v) 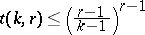 [a3]. The situation of small
[a3]. The situation of small 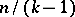 has been studied extensively, as have been the cases
has been studied extensively, as have been the cases  . The case of small
. The case of small 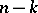 is also well-studied; this leads to the covering number. See [a5] for details.
is also well-studied; this leads to the covering number. See [a5] for details.
References
| [a1] | D. de Caen, "Extension of a theorem of Moon and Moser on complete subgraphs" Ars Combinatoria , 16 (1983) pp. 5–10 |
| [a2] | G. Katona, T. Nemetz, M. Simonovits, "On a graph problem of Turán" Mat. Lapok , 15 (1964) pp. 228–238 |
| [a3] | A. Sidorenko, "Systems of sets that have the 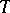 -property" Moscow Univ. Math. Bull. , 36 (1981) pp. 22–26 -property" Moscow Univ. Math. Bull. , 36 (1981) pp. 22–26 |
| [a4] | A. Sidorenko, "Upper bounds on Turán numbers" J. Combin. Th. A , 77 : 1 (1997) pp. 134–147 |
| [a5] | A. Sidorenko, "What we know and what we do not know about Turán numbers" Graphs Combin. , 11 (1995) pp. 179–199 |
| [a6] | P. Turán, "Research Problems" Magyar Tud. Akad. Mat. Kutato Internat. Közl. , 6 (1961) pp. 417–423 |
Turán number. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tur%C3%A1n_number&oldid=23548