Nikodým convergence theorem
A theorem [a6], [a7], [a4] saying that for a pointwise convergent sequence  of countably additive measures (cf. Measure) defined on a
of countably additive measures (cf. Measure) defined on a  -algebra
-algebra  , i.e.,
, i.e.,  ,
,  :
:
i) the limit  is a countably additive measure;
is a countably additive measure;
ii)  is uniformly
is uniformly  -additive. As is well-known, the Nikodým convergence theorem for measures fails in general for algebras of sets. But there are convergence theorems in which the initial convergence conditions are imposed on certain subfamilies of a given
-additive. As is well-known, the Nikodým convergence theorem for measures fails in general for algebras of sets. But there are convergence theorems in which the initial convergence conditions are imposed on certain subfamilies of a given 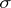 -algebra; those subfamilies need not be
-algebra; those subfamilies need not be 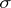 -algebras. The following definitions are useful [a2], [a9], [a8]:
-algebras. The following definitions are useful [a2], [a9], [a8]:
SCP) An algebra 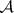 has the sequential completeness property if each disjoint sequence
has the sequential completeness property if each disjoint sequence 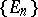 from
from 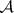 has a subsequence
has a subsequence 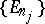 whose union is in
whose union is in 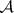 .
.
SIP) An algebra  has the subsequentional interpolation property if for each subsequence
has the subsequentional interpolation property if for each subsequence 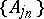 of each disjoint sequence
of each disjoint sequence 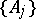 from
from 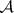 there are a subsequence
there are a subsequence 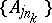 and a set
and a set 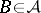 such that
such that
 |
and 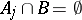 for
for 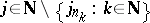 .
.
The Nikodým convergence theorem holds on algebras with SCP) and SIP).
A famous result of J. Dieudonné [a3], Prop. 8, and A. Grothendieck [a5], p. 150, states that for compact metric spaces, respectively locally compact spaces, convergence of a sequence of regular Borel measures on every open set implies convergence on all Borel sets (cf. also Borel set).
Many related results can be found in [a1], [a8], where the method of diagonal theorems is used instead of the commonly used Baire category theorem (see [a4], [a10] and Diagonal theorem).
See also Brooks–Jewett theorem; Vitali–Hahn–Saks theorem.
References
| [a1] | P. Antosik, C. Swartz, "Matrix methods in analysis" , Lecture Notes Math. , 1113 , Springer (1985) |
| [a2] | C. Constantinescu, "Some properties of spaces of measures" Suppl. Atti Sem. Mat. Fis. Univ. Modena , 35 (1991) pp. 1–286 |
| [a3] | J. Dieudonné, "Sur la convergence des suites de mesures de Radon" An. Acad. Brasil. Ci. , 23 (1951) pp. 21–38, 277–282 |
| [a4] | N. Dunford, J.T. Schwartz, "Linear operators Part I" , Interscience (1958) |
| [a5] | A. Grothendieck, "Sur les applications linéares faiblement compactes d'espaces du type  " Canad. J. Math. , 5 (1953) pp. 129–173 " Canad. J. Math. , 5 (1953) pp. 129–173 |
| [a6] | O. Nikodym, "Sur les suites de functions parfaitement additives d'ensembles abstraits" C.R. Acad. Sci. Paris , 192 (1931) pp. 727 |
| [a7] | O. Nikodym, "Sur les suites convergentes de functions parfaitement additives d'ensembles abstraits" Monatsh. Math. , 40 (1933) pp. 427–432 |
| [a8] | E. Pap, "Null-additive set functions" , Kluwer Acad. Publ. &Ister Sci. (1995) |
| [a9] | W. Schachermayer, "On some classsical measure-theoretic theorems for non-sigma complete Boolean algebras" Dissert. Math. , 214 (1982) pp. 1–33 |
| [a10] | C. Swartz, "Introduction to functional analysis" , M. Dekker (1992) |
Nikodým convergence theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Nikod%C3%BDm_convergence_theorem&oldid=23434