Ray-Knight compactification
From Encyclopedia of Mathematics
Revision as of 18:54, 24 March 2012 by Ulf Rehmann (talk | contribs) (moved Ray–Knight compactification to Ray-Knight compactification: ascii title)
A powerful tool to study homogeneous strong Markov processes (cf. Markov process) under some general hypotheses. The idea is to imbed as a set the state space 
of the process in a compact metrizable space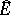 
such that the resolvent 
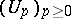of the transition semi-group 
(cf. Transition-operator semi-group) has a unique extension to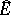 
as a resolvent 
with nice analytical properties. This Ray resolvent is associated to a semi-group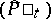 
(
need not be the identity: existence of branching points), quite indistinguishable from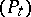 
on 
. The Ray–Knight compactification allows one to extend easily numerous important results for Feller processes (cf. Feller process) to strong Markov processes, to define entrance boundaries, etc.
References
| [a1] | C. Dellacherie, P.A. Meyer, "Probabilities and potential" , C , North-Holland (1988) pp. Chapt. XII (Translated from French) |
| [a2] | R.K. Getoor, "Markov processes: Ray processes and right processes" , Lect. notes in math. , 440 , Springer (1975) |
| [a3] | M.J. Sharpe, "General theory of Markov processes" , Acad. Press (1988) |
How to Cite This Entry:
Ray-Knight compactification. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ray-Knight_compactification&oldid=22967
Ray-Knight compactification. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ray-Knight_compactification&oldid=22967
This article was adapted from an original article by C. Dellacherie (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article