Men'shov-Rademacher theorem

A theorem on the almost-everywhere convergence of orthogonal series: If a system of functions is orthonormal on a segment and if

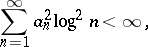then the series

(*)

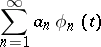converges almost-everywhere on 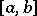. This result has been proved independently by D.E. Men'shov [1] and H. Rademacher [2]. Men'shov showed also that this assertion is sharp in the following sense. If a monotone increasing sequence satisfies the condition 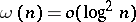, then one can find an orthogonal series (*), diverging everywhere, the coefficients of which satisfy the condition

References

[1]	D.E. Men'shov, "Sur la séries de fonctions orthogonales (I)" Fund. Math. , 4 (1923) pp. 82–105
[2]	H. Rademacher, "Einige Sätze über Reihen von allgemeinen Orthogonalfunktionen" Math. Ann. , 87 (1922) pp. 112–138
[3]	G. Alexits, "Konvergenzprobleme der Orthogonalreihen" , Deutsch. Verlag Wissenschaft. (1960)

References

[a1]	A. Zygmund, "Trigonometric series" , 2 , Cambridge Univ. Press (1988)