Gleason-Kahane-Żelazko theorem

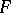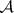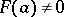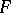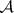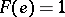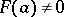Let be a non-zero linear and multiplicative functional on a complex Banach algebra with a unit , and let denote the set of all invertible elements of . Then , and for any one has . A.M. Gleason [a1] and, independently, J.P. Kahane and W. Żelazko [a5], [a6] proved that the property characterizes multiplicative functionals: If is a linear functional on a complex unital Banach algebra such that and for 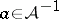, then 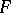is multiplicative. Equivalently: a linear functional on a commutative complex unital Banach algebra 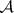is multiplicative if and only if 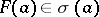for all 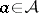, where stands for the spectrum of (cf. also Spectrum of an element). As there is a one-to-one correspondence between linear multiplicative functionals and maximal ideals, the theorem can also be phrased in the following way: A codimension-one subspace 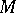of a commutative complex unital Banach algebra 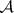is an ideal if and only if each element of is contained in a non-trivial ideal. The theorem is not valid for real Banach algebras.

The Gleason–Kahane–Żelazko theorem has been extended into several directions:

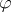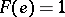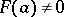1) If is non-constant entire function and is a linear functional on a complex unital Banach algebra , such that and for 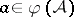, then 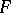is multiplicative [a3].

2) Let 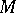be a finite-codimensional subspace of the algebra of all continuous complex-valued functions on a compact space 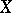. If each element of 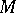is equal to zero at some point of 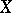, then the functions from 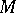have a common zero in 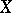[a2]. It is not known if the analogous result is valid for all commutative unital Banach algebras.

3) The assumption of linearity of the functional 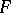has been weakened, and the result has been extended to mappings between Banach and topological algebras.

See [a4] for more information about the history, related problems, and further references.

References

[a1]	A.M. Gleason, "A characterization of maximal ideals" J. d'Anal. Math. , 19 (1967) pp. 171–172
[a2]	K. Jarosz, "Finite codimensional ideals in function algebras" Trans. Amer. Math. Soc. , 287 : 2 (1985) pp. 779–785
[a3]	K. Jarosz, "Multiplicative functionals and entire functions II" Studia Math. , 124 : 2 (1997) pp. 193–198
[a4]	K. Jarosz, "When is a linear functional multiplicative?" , Function Spaces: Proc. 3rd Conf. Function Spaces , Contemp. Math. , 232 , Amer. Math. Soc. (1999)
[a5]	J.-P. Kahane, W. Żelazko, "A characterization of maximal ideals in commutative Banach algebras" Studia Math. , 29 (1968) pp. 339–343
[a6]	W. Żelazko, "A characterization of multiplicative linear functionals in complex Banach algebras" Studia Math. , 30 (1968) pp. 83–85