Banach-Jordan algebra

Jordan–Banach algebra

A Jordan algebra over the field of real or complex numbers, endowed with a complete norm 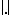satisfying

for all , 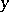in the algebra. Since an (associative) Banach algebra is a Banach–Jordan algebra under the Jordan product 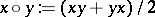, the theory of Banach–Jordan algebras can be regarded as a generalization of that of Banach algebras. For forerunners in this last theory, see Banach algebra and [a5]. Pioneering papers on Banach–Jordan algebras are [a4], [a19] and [a13]. A relatively complete panoramic view of the results on Banach–Jordan algebras can be obtained by combining [a16], [a3] and [a7].

Spectral methods in Banach–Jordan algebras have been possible thanks to the concept of invertible element in a Jordan algebra with a unit, introduced by N. Jacobson and K. McCrimmon (see [a12] or Jordan algebra). From this concept, the spectrum of an arbitrary element of a Banach–Jordan algebra is defined as in the associative case, and the spectral radius formula holds. In fact, Banach–Jordan algebras are "locally spectrally" associative. 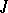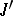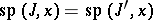This means that each element in such an algebra can be imbedded in some closed associative subalgebra of satisfying for every 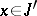. Then, for a single element in a complex Banach–Jordan algebra, a holomorphic functional calculus follows easily.

A Jordan algebra is said to be semi-simple (or semi-primitive, as preferred by people working in pure algebra) whenever its Jacobson-type radical [a11] is zero (cf. also Jacobson radical). Refining spectral methods, B. Aupetit [a2] gave a Jacobson-representation-theory-free proof of Johnson's uniqueness-of-norm theorem for semi-simple Banach algebras, and extended the result to semi-simple Banach–Jordan algebras. The absence of representation theory in Aupetit's proof was relevant because, although semi-simple Jordan algebras can be expressed as subdirect products of Jordan algebras which are "primitive" (in a peculiar Jordan sense), primitive Jordan algebras were not well-understood at that time. Aupetit's methods have shown also useful in extending from Banach algebras to Banach–Jordan algebras many other relevant results (see again [a3]), as well as in obtaining a general non-associative variant of Johnson's theorem [a15]. Recently, using work of E.I. Zel'manov [a22] on Jordan algebras without any finiteness condition, primitive Banach–Jordan algebras have been described in detail [a8]. Such a description has allowed one to extend to Banach–Jordan algebras the Johnson–Sinclair theorem, stating that derivations on semi-simple Banach algebras (cf. also Derivation in a ring) are automatically continuous [a18].

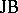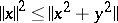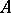-algebras are defined as the real Banach–Jordan algebras satisfying for all . The basic theory of -algebras, originally due to E.M. Alfsen, F.W. Shultz and E. Stormer [a1], is fully treated in [a10]. If is a -algebra, then the self-adjoint part of is a 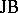-algebra under the Jordan product. Closed subalgebras of 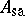, for some 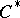-algebra 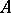, become relevant examples of 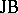-algebras, and are called 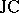-algebras. Through the consideration of 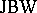-algebras (i.e., 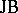-algebras that are dual Banach spaces, cf. also Banach space), 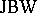-factors (i.e., prime 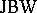-algebras), and factor representations of a given 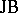-algebra 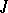(i.e., -dense range homomorphisms from 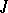to 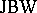-factors), the knowledge of arbitrary 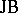-algebras is reasonably reduced to that of -algebras and the exceptional -algebra 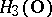of all Hermitian -matrices over the alternative division algebra of real octonions.

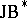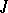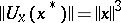-algebras are defined as complex Banach–Jordan algebras endowed with a conjugate-linear algebra involution satisfying for every 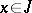. Here, for , 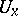denotes the operator on 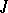defined by 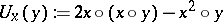for every 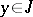. Every 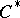-algebra becomes a 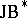-algebra under its Jordan product. -algebras are closely related to -algebras. Indeed, -algebras are nothing but the self-adjoint parts of 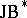-algebras [a20]. 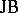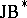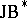The one-to-one categorical correspondence between -algebras and -algebras derived from the above result completely reduces the -theory of -algebras to the theory of 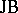-algebras. However, 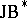-algebras are of interest on their own, mainly due to their connection with complex analysis (see [a6], [a17], and [a21]). 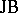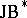Using Zel'manov's prime theorem, the structure theory of - and -algebras can be refined as follows (see [a9]). A 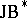-algebra 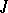is primitive if and only if it is of one of the following types:

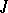is the unique 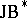-algebra whose self-adjoint part is .

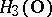There exists a complex Hilbert space 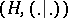of dimension 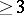, with a conjugation 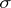and a -invariant norm-one element 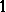, such that 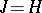as complex vector spaces, whereas the product , the involution , and the norm 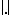of 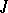are given by

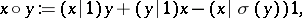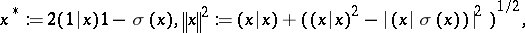respectively.

There exists a primitive 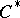-algebra 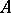such that 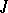is a closed self-adjoint Jordan subalgebra of the -algebra , of multipliers of , containing .

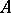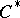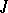There exists a primitive -algebra with a -involution such that is a closed self-adjoint Jordan subalgebra of 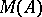contained in the -Hermitian part of 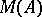and containing the -Hermitian part of .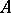

From the point of view of analysis, the Jordan identity 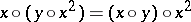(which, together with the commutativity, is characteristic of Jordan algebras) can be regarded as a theorem instead of as an axiom. Indeed, if a unital complete normed non-associative complex algebra 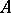is subjected to the geometric conditions that, through the Vidav–Palmer theorem, characterize 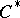-algebras in the associative setting, then 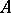under the product and a suitable involution becomes a 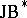-algebra [a14].

This article is dedicated to the memory of Eulalia Garcia Rus.

References

[a1]	E.M. Alfsen, F.W. Shultz, E. Stormer, "A Gelfand–Neumark theorem for Jordan algebras" Adv. Math. , 28 (1978) pp. 11–56
[a2]	B. Aupetit, "The uniqueness of the complete norm topology in Banach algebras and Banach Jordan algebras" J. Funct. Anal. , 47 (1982) pp. 7–25
[a3]	B. Aupetit, "Recent trends in the field of Jordan–Banach algebras" J. Zemánek (ed.) , Functional Analysis and Operator Theory , 30 , Banach Center Publ. (1994) pp. 9–19
[a4]	V.K. Balachandran, P.S. Rema, "Uniqueness of the complete norm topology in certain Banach Jordan algebras" Publ. Ramanujan Inst. , 1 (1969) pp. 283–289
[a5]	F.F. Bonsall, J. Duncan, "Complete normed algebras" , Springer (1973)
[a6]	R.B. Braun, W. Kaup, H. Upmeier, "A holomorphic characterization of Jordan 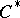-algebras" Math. Z. , 161 (1978) pp. 277–290
[a7]	M. Cabrera, A. Moreno, A. Rodriguez, "Normed versions of the Zel'manov prime theorem: positive results and limits" A. Gheondea (ed.) R.N. Gologan (ed.) D. Timotin (ed.) , Operator Theory, Operator Algebras and Related Topics (16th Internat. Conf. Operator Theory, Timisoara (Romania) July, 2-10, 1996) , The Theta Foundation, Bucharest (1997) pp. 65–77
[a8]	M. Cabrera, A. Moreno, A. Rodriguez, "Zel'manov's theorem for primitive Jordan–Banach algebras" J. London Math. Soc. , 57 (1998) pp. 231–244
[a9]	A. Fernandez, E. Garcia, A. Rodriguez, "A Zelmanov prime theorem for 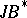-algebras" J. London Math. Soc. , 46 (1992) pp. 319–335
[a10]	H. Hanche-Olsen, E. Stormer, "Jordan operator algebras" , Monograph Stud. Math. , 21 , Pitman (1984)
[a11]	L. Hogben, K. Mccrimmon, "Maximal modular inner ideals and the Jacobson radical of a Jordan algebra" J. Algebra , 68 (1981) pp. 155–169
[a12]	N. Jacobson, "Structure and representations of Jordan algebras" , Colloq. Publ. , 37 , Amer. Math. Soc. (1968)
[a13]	P.S. Putter, B. Yood, "Banach Jordan -algebras" Proc. London Math. Soc. , 41 (1980) pp. 21–44
[a14]	A. Rodriguez, "Nonassociative normed algebras spanned by hermitian elements" Proc. London Math. Soc. , 47 (1983) pp. 258–274
[a15]	A. Rodriguez, "The uniqueness of the complete algebra norm topology in complete normed nonassociative algebras" J. Funct. Anal. , 60 (1985) pp. 1–15
[a16]	A. Rodriguez, "Jordan structures in analysis" W. Kaup (ed.) K. McCrimmon (ed.) H.P. Petersson (ed.) , Jordan Algebras (Proc. Conf. Oberwolfach, Germany, August, 9-15, 1992) , de Gruyter (1994) pp. 97–186
[a17]	H. Upmeier, "Symmetric Banach manifolds and Jordan 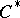-algebras" , North-Holland (1985)
[a18]	A.R. Villena, "Continuity of derivations on Jordan–Banach algebras." Studia Math. , 118 (1996) pp. 205–229
[a19]	C. Viola Devapakkiam, "Jordan algebras with continuous inverse" Math. Japon. , 16 (1971) pp. 115–125
[a20]	J.D. M. Wright, "Jordan 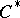-algebras" Michigan Math. J. , 24 (1977) pp. 291–302
[a21]	M.A. Youngson, "Non unital Banach Jordan algebras and 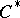-triple systems" Proc. Edinburgh Math. Soc. , 24 (1981) pp. 19–31
[a22]	E. Zel'manov, "On prime Jordan algebras II" Sib. Math. J. , 24 (1983) pp. 89–104