Rational representation
of an algebraic group 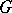 over an algebraically closed field
over an algebraically closed field 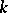
A linear representation of  on a finite-dimensional vector space
on a finite-dimensional vector space 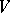 over
over 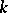 which is a rational homomorphism of
which is a rational homomorphism of  into
into 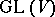 . One also says that
. One also says that  is a rational
is a rational 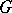 -module. Direct sums and tensor products of a finite number of rational representations of
-module. Direct sums and tensor products of a finite number of rational representations of  are rational representations. Subrepresentations and quotient representations of any rational representation are rational representations. Symmetric and exterior powers of any rational representation are rational representations. The representation contragredient to a rational representation is a rational representation.
are rational representations. Subrepresentations and quotient representations of any rational representation are rational representations. Symmetric and exterior powers of any rational representation are rational representations. The representation contragredient to a rational representation is a rational representation.
If 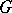 is finite, then each of its linear representations will be a rational representation, and the theory of rational representations coincides with the theory of representations of finite groups (cf. Representation of a group). To a large extent, specific methods of the theory of linear algebraic groups are used to study rational representations in case the group under consideration is connected, and the most thoroughly developed theory is that of rational representations of connected semi-simple algebraic groups. Let
is finite, then each of its linear representations will be a rational representation, and the theory of rational representations coincides with the theory of representations of finite groups (cf. Representation of a group). To a large extent, specific methods of the theory of linear algebraic groups are used to study rational representations in case the group under consideration is connected, and the most thoroughly developed theory is that of rational representations of connected semi-simple algebraic groups. Let 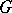 be such a group,
be such a group, 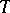 a maximal torus,
a maximal torus, 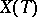 its group of rational characters (written additively),
its group of rational characters (written additively), 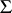 the root system of
the root system of 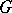 with respect to
with respect to 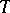 ,
,  its Weyl group, and
its Weyl group, and 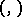 a
a 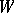 -invariant positive-definite non-degenerate scalar product on
-invariant positive-definite non-degenerate scalar product on 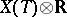 . Now let
. Now let 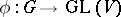 be a rational representation. The restriction of
be a rational representation. The restriction of 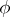 to
to 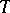 decomposes into a direct sum of one-dimensional representations; more precisely,
decomposes into a direct sum of one-dimensional representations; more precisely,
 |
where  is some set of characters of
is some set of characters of  , called the weights of the representation, and
, called the weights of the representation, and
 |
The set of weights 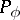 is invariant under the action of
is invariant under the action of 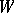 .
.
If  , then every rational representation of
, then every rational representation of 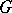 is completely reducible, but if
is completely reducible, but if 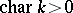 , then this is not so (see Mumford hypothesis). Whatever the characteristic of
, then this is not so (see Mumford hypothesis). Whatever the characteristic of 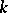 , however, there is a complete description of the irreducible rational representations.
, however, there is a complete description of the irreducible rational representations.
Let 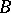 be a Borel subgroup in
be a Borel subgroup in 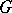 containing
containing 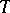 and let
and let 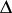 be the set of simple roots in
be the set of simple roots in 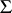 defined by
defined by 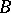 . Identify the group
. Identify the group 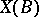 of rational characters of
of rational characters of 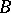 with
with 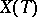 . In the space
. In the space 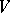 , for any irreducible rational representation
, for any irreducible rational representation 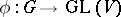 there is a unique one-dimensional weight subspace
there is a unique one-dimensional weight subspace 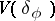 ,
, 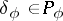 , invariant under
, invariant under 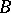 . The character
. The character 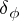 is called the highest weight of the irreducible rational representation
is called the highest weight of the irreducible rational representation 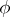 ; it is dominant, i.e.
; it is dominant, i.e.  for any
for any 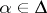 , and every other weight
, and every other weight 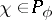 has the form
has the form
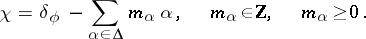 |
The mapping 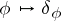 defines a bijection between the classes of equivalent irreducible rational representations and the dominant elements of
defines a bijection between the classes of equivalent irreducible rational representations and the dominant elements of  . An explicit construction of all irreducible rational representations can be obtained in the following way. Let
. An explicit construction of all irreducible rational representations can be obtained in the following way. Let 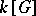 be the algebra of regular functions on
be the algebra of regular functions on 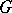 . Given any
. Given any  , consider the subspace
, consider the subspace
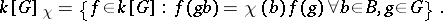 |
It is finite-dimensional and is a rational 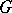 -module under the action of
-module under the action of 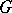 by left translation. The geometric meaning of this space is as follows: it can be canonically identified with the set of regular sections of the one-dimensional homogeneous vector bundle over
by left translation. The geometric meaning of this space is as follows: it can be canonically identified with the set of regular sections of the one-dimensional homogeneous vector bundle over 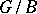 determined by the character
determined by the character  . Let
. Let 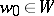 be the element mapping positive roots into negative ones. If
be the element mapping positive roots into negative ones. If 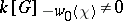 , then
, then 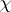 is a dominant character and the minimal non-zero
is a dominant character and the minimal non-zero 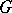 -submodule in
-submodule in 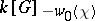 is an irreducible rational
is an irreducible rational 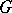 -module with highest weight
-module with highest weight  . Every irreducible rational
. Every irreducible rational 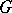 -module can be obtained in this way. If
-module can be obtained in this way. If 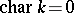 , then the
, then the 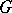 -module
-module  is itself irreducible.
is itself irreducible.
To obtain irreducible rational representations, one often applies the above-mentioned operations to given rational representations. For example, if 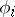 is an irreducible rational representation with highest weight
is an irreducible rational representation with highest weight 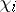 ,
, 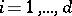 , then some quotient representation of
, then some quotient representation of 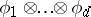 is an irreducible rational representation with highest weight
is an irreducible rational representation with highest weight 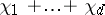 (it is called the Cartan product of
(it is called the Cartan product of 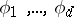 ). If
). If 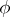 is an irreducible rational representation with highest weight
is an irreducible rational representation with highest weight 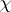 , then some quotient representation of
, then some quotient representation of 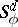 is an irreducible rational representation with highest weight
is an irreducible rational representation with highest weight 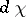 . Moreover
. Moreover 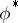 is irreducible and its highest weight is
is irreducible and its highest weight is 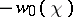 .
.
Let 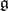 be the Lie algebra of
be the Lie algebra of 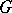 (cf. Lie algebra of an algebraic group). If
(cf. Lie algebra of an algebraic group). If 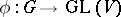 is a rational representation, then its differential
is a rational representation, then its differential 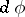 is a representation of the Lie algebra
is a representation of the Lie algebra 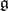 . A rational representation
. A rational representation 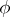 is called infinitesimally irreducible if
is called infinitesimally irreducible if 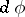 is an irreducible representation of the algebra
is an irreducible representation of the algebra 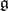 . An infinitesimally-irreducible rational representation is irreducible, and when
. An infinitesimally-irreducible rational representation is irreducible, and when 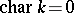 , the converse is also true (which largely reduces the theory of rational representations of a group to the theory of representations of its Lie algebra). But when
, the converse is also true (which largely reduces the theory of rational representations of a group to the theory of representations of its Lie algebra). But when  , this is not so; the infinitesimally-irreducible rational representations in this case are just those irreducible rational representations with highest weight
, this is not so; the infinitesimally-irreducible rational representations in this case are just those irreducible rational representations with highest weight 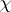 for which
for which
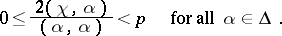 |
Moreover, all the irreducible rational representations can be constructed using the infinitesimally-irreducible ones. More precisely, if 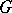 is simply connected, that is, if
is simply connected, that is, if 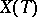 coincides with the lattice of weights of the root system
coincides with the lattice of weights of the root system  , then every irreducible rational representation factors uniquely into a tensor product of the form
, then every irreducible rational representation factors uniquely into a tensor product of the form
 |
where 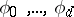 are infinitesimally irreducible, and
are infinitesimally irreducible, and 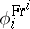 is the representation obtained by applying the Frobenius automorphism
is the representation obtained by applying the Frobenius automorphism 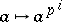 (
(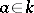 ,
, 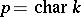 ) to the matrix entries of the representation
) to the matrix entries of the representation 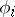 .
.
References
| [1] | A. Borel, "Linear algebraic groups" , Benjamin (1969) MR0251042 Zbl 0206.49801 Zbl 0186.33201 |
| [2] | A. Borel, "Linear representations of semi-simple algebraic groups" R. Hartshorne (ed.) , Algebraic geometry (Arcata, 1974) , Proc. Symp. Pure Math. , 29 , Amer. Math. Soc. (1975) pp. 421–440 MR0372054 Zbl 0311.20022 |
| [3] | J.E. Humphreys, "Linear algebraic groups" , Springer (1975) MR0396773 Zbl 0325.20039 |
| [4] | A. Borel (ed.) R. Carter (ed.) C.W. Curtis (ed.) N. Iwahori (ed.) T.A. Springer (ed.) R. Steinberg (ed.) , Seminar on algebraic groups and related finite groups , Lect. notes in math. , 131 , Springer (1970) Zbl 0192.36201 |
| [5] | R.G. Steinberg, "Lectures on Chevalley groups" , Yale Univ. Press (1968) MR0466335 Zbl 1196.22001 |
| [6] | R. Steinberg, "Representations of algebraic groups" Nagoya Math. J. , 22 (1963) pp. 33–56 MR0155937 Zbl 0271.20019 |
| [7] | G. Hochschild, "The structure of Lie groups" , Holden-Day (1965) MR0207883 Zbl 0131.02702 |
| [8] | J.E. Humphreys, "Introduction to Lie algebras and representation theory" , Springer (1972) MR0323842 Zbl 0254.17004 |
Comments
See [a1], especially for complications in  .
.
References
| [a1] | J.C. Jantzen, "Representations of algebraic groups" , Acad. Press (1987) MR0899071 Zbl 0654.20039 |
Rational representation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Rational_representation&oldid=21993