Subvariety, involutive
(in symplectic geometry)
Let 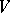 be a vector space of dimension
be a vector space of dimension  and
and 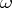 a non-degenerate alternating
a non-degenerate alternating  -form on it. Given a subspace
-form on it. Given a subspace 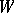 of
of  , one defines (as usual)
, one defines (as usual)
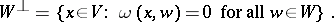 |
One says that 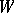 is an isotropic subspace if
is an isotropic subspace if 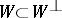 , that it is an involutive subspace (or co-isotropic subspace) if
, that it is an involutive subspace (or co-isotropic subspace) if 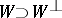 , and that it is a Lagrangian subspace if
, and that it is a Lagrangian subspace if 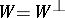 . Note that for
. Note that for 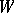 to be involutive it is necessary that
to be involutive it is necessary that 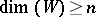 .
.
Now, let 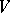 be a subvariety (possibly with singularities; or, more generally, an analytic subset) of a symplectic manifold
be a subvariety (possibly with singularities; or, more generally, an analytic subset) of a symplectic manifold 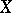 . Let
. Let 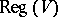 be the set of points of
be the set of points of 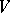 which have a neighbourhood in
which have a neighbourhood in 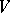 that is free of singular points. Then
that is free of singular points. Then  is an involutive subvariety of
is an involutive subvariety of 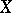 if for all
if for all 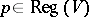 the subspace
the subspace 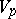 of
of 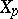 is involutive. The notions of an isotropic subvariety and a Lagrangian subvariety are defined analogously. If
is involutive. The notions of an isotropic subvariety and a Lagrangian subvariety are defined analogously. If 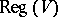 is dense in
is dense in 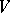 , then
, then 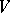 is involutive if and only if for every two
is involutive if and only if for every two 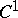 -functions
-functions 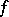 ,
, 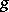 on
on 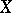 which vanish on
which vanish on 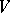 the Poisson bracket
the Poisson bracket  (defined by the symplectic
(defined by the symplectic  -form on
-form on 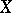 ) also vanishes on
) also vanishes on 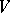 .
.
References
| [a1] | P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) (Translated from French) |
Subvariety, involutive. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Subvariety,_involutive&oldid=19173