Polydisc
From Encyclopedia of Mathematics
polycylinder
A region
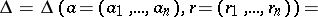 |
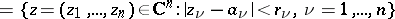 |
in a complex space  ,
,  , which is the topological product of
, which is the topological product of  discs
discs
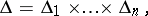 |
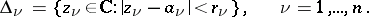 |
The point  is the centre of the polydisc
is the centre of the polydisc  ,
, 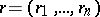 ,
,  ,
,  , is its polyradius. With
, is its polyradius. With  ,
, 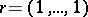 one obtains the unit polydisc. The distinguished boundary of
one obtains the unit polydisc. The distinguished boundary of  is the set
is the set
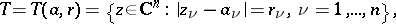 |
which is a part of its complete topological boundary 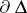 . A polydisc is a complete Reinhardt domain.
. A polydisc is a complete Reinhardt domain.
A natural generalization of the concept of a polydisc is that of a polyregion (polycircular region, generalized polycylinder) 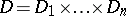 , which is the topological product of, in general multiply-connected, regions
, which is the topological product of, in general multiply-connected, regions 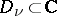 ,
,  . The boundary
. The boundary  of a polyregion
of a polyregion 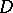 consists of
consists of  sets of dimension
sets of dimension 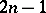 :
:
 |
the common part of which is the  -dimensional distinguished boundary of
-dimensional distinguished boundary of 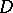 :
:
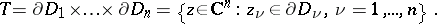 |
Comments
References
| [a1] | W. Rudin, "Function theory in polydiscs" , Benjamin (1969) |
How to Cite This Entry:
Polydisc. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Polydisc&oldid=19171
Polydisc. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Polydisc&oldid=19171
This article was adapted from an original article by E.D. Solomentsev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article