Fine topology

in potential theory

The weakest topology in which all superharmonic functions on 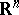are continuous. Objects related to the fine topology are described as "fine" , "finely" , etc.

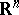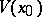The notion of fine topology is closely connected with that of a thin set (cf. also Thinness of a set). The fine topology is stronger that the usual Euclidean topology on , i.e. every Euclidean-open set is finely open. A fine neighbourhood of a point is a set such that 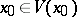and such that the complement 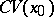is a thin set at . The finely-open sets are unions of pre-images under mappings by superharmonic functions of the extended real line and of intervals of the form 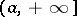, , 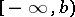, 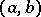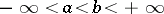. Every superharmonic function on an open set 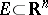is finely continuous on 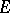. 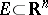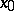A set is thin at a point if and only if is a finely-isolated point of .

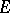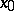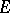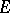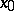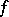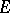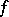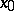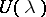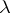Let be a fine-limit point of , that is, is not thin at , and let be a function defined on . The number is called the fine limit of at if for every neighbourhood of in 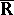there exists a fine neighbourhood 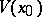of 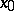such that

If 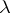is the fine limit of 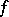at , then there exists a fine neighbourhood 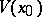such that 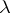is an ordinary limit at 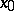of the restriction 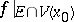(Cartan's theorem).

Let 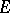be a closed set, thin at a point 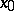, and let 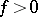be a superharmonic function defined on 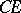in a neighbourhood of 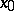. Then 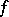has a fine limit at .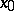

A fine topology has also been constructed in axiomatic potential theory (cf. [3]).

References

[1]	M. Brélot, "Eléments de la théorie classique du potentiel" , Sorbonne Univ. Centre Doc. Univ. , Paris (1959)
[2]	N.S. Landkof, "Foundations of modern potential theory" , Springer (1972) (Translated from Russian)
[3]	M. Brélot, "Lectures on potential theory" , Tata Inst. (1960)

Comments

A potential theory for finely-harmonic and finely-hyperharmonic functions is developed in [a1]. See also [a2].

References

[a1]	B. Fuglede, "Finely harmonic functions" , Springer (1972)
[a2]	J. Lukeš, J. Malý, L. Zajíček, "Fine topology methods in real analysis and potential theory" , Springer (1986)