Distributions, complete family of
A family of probability measures 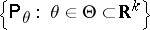 , defined on a measure space
, defined on a measure space 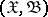 , for which the unique unbiased estimator of zero in the class of
, for which the unique unbiased estimator of zero in the class of  -measurable functions on
-measurable functions on 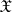 is the function identically equal to zero, that is, if
is the function identically equal to zero, that is, if  is any
is any 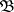 -measurable function defined on
-measurable function defined on 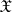 satisfying the relation
satisfying the relation
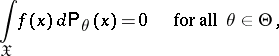 | (*) |
then 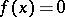
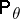 -almost-everywhere, for all
-almost-everywhere, for all 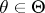 . For example, a family of exponential distributions is complete. If the relation (*) is satisfied under the further assumption that
. For example, a family of exponential distributions is complete. If the relation (*) is satisfied under the further assumption that 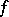 is bounded, then the family
is bounded, then the family 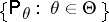 is said to be boundedly complete. Boundedly-complete families of distributions of sufficient statistics play a major role in mathematical statistics, in particular in the problem of constructing similar tests (cf. Similar test) with a Neyman structure.
is said to be boundedly complete. Boundedly-complete families of distributions of sufficient statistics play a major role in mathematical statistics, in particular in the problem of constructing similar tests (cf. Similar test) with a Neyman structure.
References
| [1] | Yu.V. Linnik, "Statistical problems with nuisance parameters" , Amer. Math. Soc. (1968) (Translated from Russian) |
| [2] | E.L. Lehmann, "Testing statistical hypotheses" , Wiley (1959) |
Comments
References
| [a1] | S. Zacks, "The theory of statistical inference" , Wiley (1971) |
Distributions, complete family of. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Distributions,_complete_family_of&oldid=19001