Arcsine distribution
A probability measure on the real line whose density is zero outside the interval  and is
and is  if
if  . The corresponding distribution function is equal to
. The corresponding distribution function is equal to  for
for  .
.
The generalized arcsine distribution is employed together with the arcsine distribution. To the generalized arcsine distribution corresponds the distribution function  with density
with density
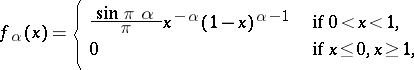 |
if  . The density
. The density  coincides with the density of the arcsine distribution. The generalized arcsine distribution is a special case of the beta-distribution. The first-order moment of the generalized arcsine distribution is
coincides with the density of the arcsine distribution. The generalized arcsine distribution is a special case of the beta-distribution. The first-order moment of the generalized arcsine distribution is 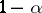 , and its variance is
, and its variance is 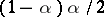 . The arcsine distribution and the generalized arcsine distribution occur in the study of the fluctuations of random walks, in renewal theory (cf. Arcsine law), and are used in mathematical statistics as special cases of the beta-distribution.
. The arcsine distribution and the generalized arcsine distribution occur in the study of the fluctuations of random walks, in renewal theory (cf. Arcsine law), and are used in mathematical statistics as special cases of the beta-distribution.
References
| [1] | W. Feller, "An introduction to probability theory and its applications" , 1–2 , Wiley (1957–1971) |
| [2] | M.G. Kendall, A. Stuart, "The advanced theory of statistics. Distribution theory" , 3. Design and analysis , Griffin (1969) |
Arcsine distribution. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Arcsine_distribution&oldid=18892