Contraction of a tensor

An operation of tensor algebra that associates with a tensor with components 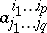, , the tensor

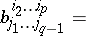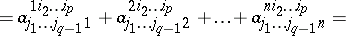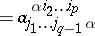(here the contraction is made with respect to the pair of indices 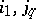). The contraction of a tensor with respect to any pair of upper and lower indices is defined similarly. The 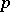-fold contraction of a tensor that is 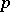-times covariant and 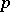-times contravariant is an invariant. Thus, the contraction of the tensor with components 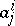is an invariant 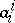, called the trace of the tensor; it is denoted by 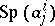, or 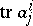. A contraction of the product of two tensors is a contraction of the product with respect to an upper index of one factor and a lower index of the other.

References

[a1]	P.K. [P.K. Rashevskii] Rashewski, "Riemannsche Geometrie und Tensoranalyse" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)