Ramanujan sums

Trigonometric sums depending on two integer parameters 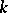and :

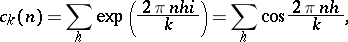when 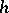runs over all non-negative integers less than 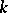and relatively prime to 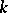. The basic properties of Ramanujan sums are multiplicity with respect to the index ,

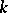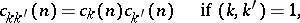and also the representation in terms of the Möbius function :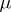

Ramanujan sums are finite if or is finite. In particular, .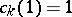

Many multiplicative functions on the natural numbers (cf. Multiplicative arithmetic function) can be expanded as series of Ramanujan sums, and, conversely, the basic properties of Ramanujan sums enable one to sum series of the form

where is a multiplicative function and is an integer. In particular,

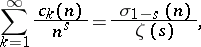where 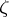is the Riemann zeta-function and 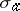is the sum of the -th powers of the divisors of . Such sums are closely connected with special series for certain additive problems in number theory (cf. Additive number theory); for example, the representation of a natural number as an even number of squares. S. Ramanujan [1] obtained many formulas involving Ramanujan sums.

References

[1]	S. Ramanujan, "On certain trigonometrical sums and their applications in the theory of numbers" Trans. Cambridge Philos. Soc. , 22 (1918) pp. 259–276 ((Also: Collected papers, Chelsea, reprint, 1962.))
[2]	G.H. Hardy, "Note on Ramanujan's trigonometrical function and certain series of arithmetical functions" Proc. Cambridge Philos. Soc. , 20 (1920–1921) pp. 263–271
[3]	G.H. Hardy (ed.) et al. (ed.) , Collected papers of S. Ramanujan , Chelsea, reprint (1962)
[4]	B. Volkmann, "Verallgemeinerung eines Satzes von Maxfield" J. Reine Angew. Math. , 271 (1974) pp. 203–213
[5]	E.C. Titchmarsh, "The theory of the Riemann zeta-function" , Clarendon Press (1951)
[6]	V.I. Levin, "The life and work of the Indian mathematician Ramanujan" Istoriko-Mat. Issled. , 13 (1960) pp. 335–378 (In Russian)