Section of a mapping
From Encyclopedia of Mathematics
A mapping 
for which 
. In a wider sense, a section of any morphism in an arbitrary category is a right-inverse morphism.
Comments
If 
is a subspace of 
, a section over 
of 
is a mapping 
such that 
for all 
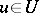. For a vector bundle 
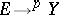, where the mapping 
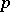is part of the structure defined, one speaks of a section of the vector bundle 
rather than of a section of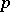 
. This applies, e.g., also to sheaves and fibrations. A standard notation for the set of sections in such a case is 
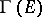, or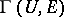 
for the set of sections of 
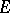over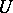 
.
How to Cite This Entry:
Section of a mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Section_of_a_mapping&oldid=18495
Section of a mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Section_of_a_mapping&oldid=18495
This article was adapted from an original article by A.F. Kharshiladze (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article