Clairaut equation
An ordinary first-order differential equation not solved with respect to its derivative:
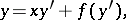 | (1) |
where  is a non-linear function. Equation (1) is named after A. Clairaut [1] who was the first to point out the difference between the general and the singular solutions of an equation of this form. The Clairaut equation is a particular case of the Lagrange equation.
is a non-linear function. Equation (1) is named after A. Clairaut [1] who was the first to point out the difference between the general and the singular solutions of an equation of this form. The Clairaut equation is a particular case of the Lagrange equation.
If  and
and 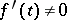 when
when  , then the set of integral curves (cf. Integral curve) of (1) consists of: a parametrically given curve
, then the set of integral curves (cf. Integral curve) of (1) consists of: a parametrically given curve
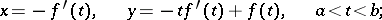 | (2) |
a one-parameter family of straight lines
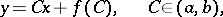 | (3) |
tangent to the curve (2); curves consisting of an arbitrary segment of the curve (2) and the two straight lines of the family (3) tangent to (2) at each end of this segment. The family (3) forms the general solution, while the curve (2), which is the envelope of the family (3), is the singular solution (see [2]). A family of tangents to a smooth non-linear curve satisfies a Clairaut equation. Therefore, geometric problems in which it is required to determine a curve in terms of a prescribed property of its tangents (common to all points of the curve) leads to a Clairaut equation.
The following first-order partial differential equation is also called a Clairaut equation:
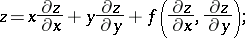 |
it has the integral
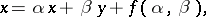 |
where 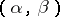 is an arbitrary point of the domain of definition of the function
is an arbitrary point of the domain of definition of the function  (see [3]).
(see [3]).
References
| [1] | A. Clairaut, Histoire Acad. R. Sci. Paris (1734) (1736) pp. 196–215 |
| [2] | V.V. Stepanov, "A course of differential equations" , Moscow (1959) (In Russian) |
| [3] | E. Kamke, "Differentialgleichungen: Lösungen und Lösungsmethoden" , 2. Partielle Differentialgleichungen  Ordnung für eine gesuchte Funktion , Akad. Verlagsgesell. (1944) Ordnung für eine gesuchte Funktion , Akad. Verlagsgesell. (1944) |
Comments
References
| [a1] | E.L. Ince, "Ordinary differential equations" , Dover, reprint (1956) |
Clairaut equation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Clairaut_equation&oldid=18469