Siegel domain

An unbounded domain in the 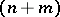-dimensional complex affine space of the form

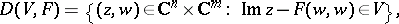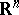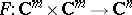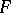where is an open convex cone in and is a mapping which is a -Hermitian form, i.e. is linear in the first argument, 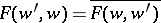, 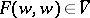(where 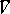is the closure of 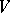), and 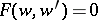only if 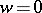. In the case 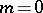(so that also 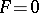) the domain 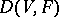is called a Siegel domain of the first kind and is simply denoted by 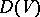; when 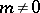, 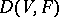is called a Siegel domain of the second kind.

The simplest example of a Siegel domain (of the first kind) is the upper half-plane in 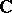. C.L. Siegel [1], in his studies on Abelian varieties, studied the domain 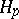in the space of complex symmetric matrices of order 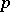, formed by matrices with positive-definite imaginary part. This domain, now known as the Siegel upper half-plane (when 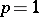it is the ordinary upper half-plane), is a Siegel domain of the first kind associated with the cone of positive-definite symmetric matrices of order . The general concept of a Siegel domain arose in connection with the theory of automorphic functions of several complex variables (see [5]). Subsequently this notion became central in the theory of homogeneous bounded domains (cf. Homogeneous bounded domain).

Any Siegel domain is analytically isomorphic to a bounded domain. For example, when 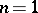a Siegel domain is isomorphic to the complex unit ball

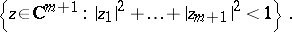Any homogeneous bounded domain is isomorphic to a Siegel domain which is homogeneous relative to affine transformations. Two Siegel domains are analytically isomorphic if and only if each can be transformed into the other by an affine transformation (see [3]).

References

[1]	C.L. Siegel, "Einführung in die Theorie der Modulfunktionen -ten Grades" Math. Ann. , 116 (1939) pp. 617–657
[2]	C.L. Siegel, "Analytic functions of several complex variables" , Princeton Univ. Press (1950)
[3]	W. Kaup, Y. Matsushima, T. Ochiai, "On the automorphisms and equivalences of generalized Siegel domains" Amer. J. Math. , 92 (1970) pp. 475–498
[4]	S. Murakami, "On automorphisms of Siegel domains" , Lect. notes in math. , 286 , Springer (1972)
[5]	S.G. Gindikin, "Algebraic problems of the theory of functions of several complex variables" Itogi Nauk. Mat. Anal. 1963 (1965) pp. 81–124 (In Russian)

Comments

General Siegel domains were introduced by I.I. Pyatetskii-Shapiro in the late 1950's (cf. [a1]). He has found an example of a homogeneous Siegel domain which is not symmetric.

References

[a1]	I.I. [I.I. Pyatetskii-Shapiro] Piatetski-Shapiro, "Automorphic functions and the geometry of classical domains" , Gordon & Breach (1969) (Translated from Russian)