Dimension polynomial

of an extension of differential fields

A polynomial describing the number of derivative constants in the solution of a system of partial differential equations; it is an analogue of the Hilbert polynomial.

Let be a differential extension of a differential field . A maximal subset of 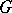that is differentially separably independent over 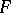is called a differential inseparability basis. A differential inseparability basis of an extension 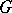over 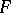that is differentially algebraically independent over 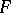is called a differential transcendence basis.

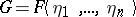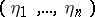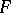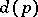Let be a finitely-generated differential extension, , and let be a generic zero of a prime differential ideal . The differential transcendence degree of over is called the differential dimension of (it is denoted by ). If 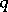is another prime differential ideal with 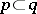, then 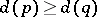and, moreover, equality can occur even for a strict inclusion. For this reason, it is desirable to have a finer measure for measuring ideals.

A filtration of the ring 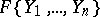of differential polynomials by the degrees of the derivations 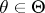induces a filtration of the extension fields 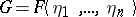of :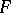

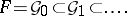There exists (see [2]) a polynomial whose value at points 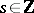for all 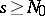is equal to the transcendence degree of the extension 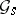of 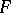. It is called the dimension polynomial of the extension , and has the form

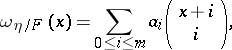where 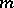is the cardinality of the set of derivation operators 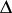and the 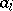are integers. The dimension polynomial is a birational invariant of the field, that is, 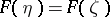implies 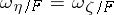, but it is not a differential birational invariant, that is, does not in general imply that 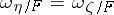. Nevertheless, this polynomial includes differential birational invariants, such as the degree 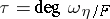of the polynomial (called the differential type of the extension 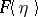over ), and the leading coefficient (called the typical differential dimension). Among the differential dimension polynomials corresponding to various systems of differential generators of a differential extension there exists a minimal one with respect to some order relation on the set of all numerical polynomials, which is hence a differential birational invariant of the extension.

The differential dimension polynomial is also defined for differential modules.

The dimension polynomial has been computed for the extensions given by the following systems (see [1], where the dimension polynomial is called the measure of rigidity of the system of equations of a field): 1) the wave equation; 2) the Maxwell equation for empty space; 3) the equation of the electromagnetic field given by potentials; and 4) the Einstein equation for empty space. Other examples of the computation of dimension polynomials can be found in [3].

References

[1]	A. Einstein, "The meaning of relativity" , Princeton Univ. Press (1953)
[2]	E.R. Kolchin, "Differential algebra and algebraic groups" , Acad. Press (1973)
[3]	A.V. Mikhalev, E.V. Pankrat'ev, "The differential dimension polynomial of a system of differential equations" , Algebra , Moscow (1980) pp. 57–67 (In Russian)

Comments

For the notions of differential separable independence, differential transcendence degree and differential algebraic independence cf. Extension of a differential field.