Residuated mapping
An isotone mapping  from a partially ordered set
from a partially ordered set  into a partially ordered set
into a partially ordered set  for which there is an isotone mapping
for which there is an isotone mapping  from
from  into
into  such that
such that  for all
for all  and
and  for all
for all 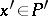 . If
. If  and
and 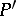 are complete lattices, then this is equivalent to the equality:
are complete lattices, then this is equivalent to the equality:
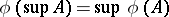 |
for every subset 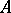 of
of 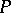 . The set of residuated mappings of a partially ordered set
. The set of residuated mappings of a partially ordered set  into itself forms a semi-group which can be partially ordered (see Ordered semi-group) by setting
into itself forms a semi-group which can be partially ordered (see Ordered semi-group) by setting  if
if 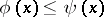 for all
for all  . The properties of this partially ordered semi-group are closely linked to the properties of the partially ordered set
. The properties of this partially ordered semi-group are closely linked to the properties of the partially ordered set 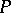 (see Lattice).
(see Lattice).
Comments
The mapping 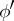 appearing in the definition is called the residual of
appearing in the definition is called the residual of  ; it is uniquely determined by
; it is uniquely determined by  . A more symmetric terminology, borrowed from category theory, calls
. A more symmetric terminology, borrowed from category theory, calls 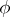 a left adjoint and
a left adjoint and 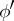 a right adjoint (see Adjoint functor). For the antitone analogues of residuated mappings see Galois correspondence.
a right adjoint (see Adjoint functor). For the antitone analogues of residuated mappings see Galois correspondence.
References
| [a1] | T.S. Blyth, M.F. Janowitz, "Residuation theory" , Pergamon (1972) |
Residuated mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Residuated_mapping&oldid=17933