Content
A set 
has (Lebesgue) content zero if for all 
there is a finite set of closed rectangles 
such that 
and 
, where 
is Lebesgue measure.
More generally, let 
be a space equipped with a ring 
of subsets such that 
(
need not be a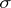 
-ring and 
need not be in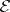 
). Let a function 
on 
be given such that 
for all 
, 
for at least one 
and such that 
is additive on 
. Such a function is called a content, and 
is the content of 
.
Define a rectangle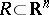 
as a product 
, where the 
are bounded closed, open or half-closed intervals, and let 
, where 
is the length of the interval 
. Define an elementary set in 
to be a finite union of rectangles. Let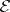 
be the collection of all elementary sets. Each 
can be written as a finite disjoint union of rectangles 
; then define 
. This defines a content on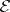 
called Jordan content.
Given a content 
on 
and any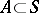 
, 
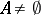, one defines
 |
where the infimum is taken over all finite sums such that 
, 
; also one sets 
. This defines an outer measure on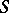 
.
References
| [a1] | J.F. Randolph, "Basic real and abstract analysis" , Acad. Press (1968) |
| [a2] | M.M. Rao, "Measure theory and integration" , Interscience (1987) |
Content. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Content&oldid=17904