Jensen formula
A relation connecting the values of a meromorphic function inside a disc with its boundary values on the circumference and with its zeros and poles. Let 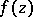 be a meromorphic function in the disc
be a meromorphic function in the disc 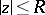 ; let
; let 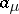 ,
,  , and
, and 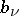 ,
, 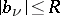 , be all the zeros and poles of
, be all the zeros and poles of 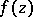 , respectively, where each pole or zero is counted as many times as its order or multiplicity. If
, respectively, where each pole or zero is counted as many times as its order or multiplicity. If  , then Jensen's formula holds:
, then Jensen's formula holds:
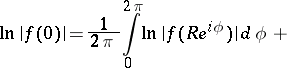 | (1) |
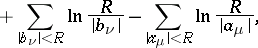 |
in which the sums extend over all zeros and poles of 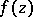 inside the disc
inside the disc 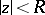 ; formula (1) was obtained by J.L. Jensen in [1]. A small modification is necessary to adapt (1) to the case
; formula (1) was obtained by J.L. Jensen in [1]. A small modification is necessary to adapt (1) to the case 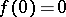 .
.
A more general formula holds, called by R. Nevanlinna the Poisson–Jensen formula, giving the values of 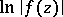 at an arbitrary point
at an arbitrary point  other than a zero or a pole:
other than a zero or a pole:
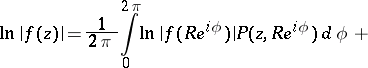 | (2) |
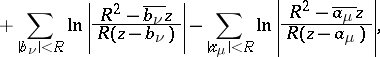 |
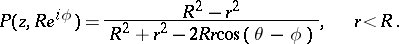 |
Formula (2) can be regarded as a generalization of the Poisson integral for a disc. Generalization of the Schwarz integral for the disc in exactly the same way gives the Schwarz–Jensen formula:
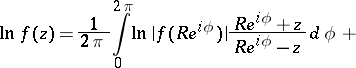 | (3) |
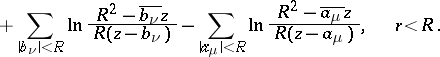 |
Formulas of the type (1)–(3) can be constructed for half-planes and other domains. The formulas (1)–(3) play an important part in value-distribution theory.
A wide generalization of the formulas (1)–(3) has been obtained by M.M. Dzhrbashyan in his theory of classes of meromorphic functions (see [3]). He succeeded in obtaining a whole family of such formulas, depending on a certain continuous parameter 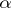 ,
,  , that is connected with an integro-differential operator
, that is connected with an integro-differential operator 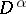 ; for example, formula (3) turns out to be the special case
; for example, formula (3) turns out to be the special case 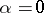 .
.
Formulas (1) and (2) can be generalized for subharmonic functions 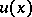 in a ball
in a ball 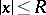 in a Euclidean space
in a Euclidean space 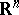 ,
, 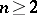 , as follows:
, as follows:
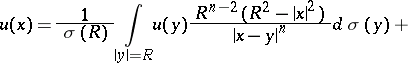 | (4) |
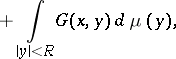 |
where 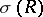 is the area of the sphere
is the area of the sphere 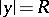 in
in 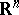 ,
, 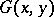 is the Green function for the ball
is the Green function for the ball 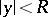 with pole at
with pole at  , and
, and 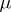 is the positive measure associated with the subharmonic function
is the positive measure associated with the subharmonic function 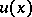 . The first summand in (4) is the least harmonic majorant of
. The first summand in (4) is the least harmonic majorant of 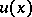 in the ball
in the ball  , expressed in the form of a Poisson integral over the boundary values; the second summand is a Green potential, which reduces in special cases to the logarithm of the modulus of the Blaschke product figuring in (2). Formula (2) is obtained from (4), taking into account that for a meromorphic function
, expressed in the form of a Poisson integral over the boundary values; the second summand is a Green potential, which reduces in special cases to the logarithm of the modulus of the Blaschke product figuring in (2). Formula (2) is obtained from (4), taking into account that for a meromorphic function 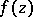 ,
, 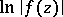 is the difference of two subharmonic functions; formula (4) is applicable to functions of this type.
is the difference of two subharmonic functions; formula (4) is applicable to functions of this type.
Now let 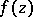 be a holomorphic function of several complex variables
be a holomorphic function of several complex variables 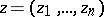 ,
, 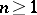 , in a closed polydisc
, in a closed polydisc
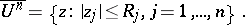 |
Of great importance also is the Jensen inequality, which can be deduced easily from the properties of plurisubharmonic functions (cf. Plurisubharmonic function), and which for 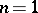 follows immediately from formula (2):
follows immediately from formula (2):
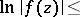 | (5) |
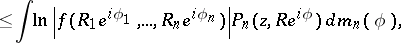 |
where
 |
is the Poisson kernel for 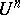 , and
, and 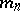 is the normalized Haar measure on the distinguished boundary
is the normalized Haar measure on the distinguished boundary
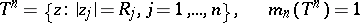 |
(see [5], [6]). Inequality (5), and certain higher-dimensional analogues of formula (2), find application in modern higher-dimensional value-distribution theory, see [7].
References
| [1] | J.L. Jensen, "Sur un nouvel et important théorème de la théorie des fonctions" Acta Math. , 22 (1899) pp. 359–364 |
| [2] | R. Nevanilinna, "Analytic functions" , Springer (1970) (Translated from German) |
| [3] | M.M. Dzhrbashyan, "Integral transforms and representation of functions in the complex domain" , Moscow (1966) (In Russian) |
| [4] | I.I. Privalov, "Subharmonic functions" , Moscow-Leningrad (1937) (In Russian) |
| [5] | V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian) |
| [6] | R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965) |
| [7] | P. Griffiths, J. King, "Nevanlinna theory and holomorphic mappings between algebraic manifolds" Acta Math. , 130 (1973) pp. 145–220 |
Comments
For a generalization of Jensen's formula to sectors and for connections with functions of regular growth and distribution of zeros, see [a3]. For higher-dimensional versions and applications, see also [a1], [a2], [a4].
References
| [a1] | P.A. Griffith, "Entire holomorphic mappings in one and several complex variables" , Annals Math. Studies , 85 , Princeton Univ. Press (1976) |
| [a2] | P. Lelong, L. Gruman, "Entire functions of several complex variables" , Springer (1986) |
| [a3] | B.Ya. Levin, "Distribution of zeros of entire functions" , Amer. Math. Soc. (1980) (Translated from Russian) |
| [a4] | L.I. Ronkin, "Inroduction to the theory of entire functions of several variables" , Transl. Math. Monogr. , 44 , Amer. Math. Soc. (1974) (Translated from Russian) |
| [a5] | W.K. Hayman, P.B. Kennedy, "Subharmonic functions" , 1 , Acad. Press (1976) |
Jensen formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Jensen_formula&oldid=17842