Double of a Riemann surface
A two-sheeted covering surface 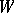 of a finite Riemann surface
of a finite Riemann surface 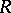 . Each interior point
. Each interior point 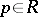 is brought into correspondence with a pair of points
is brought into correspondence with a pair of points 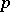 and
and 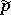 of the double
of the double 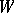 ; in other words, two conjugate points
; in other words, two conjugate points 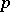 and
and  are situated over
are situated over 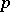 . Each point
. Each point 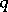 of the boundary of
of the boundary of 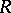 is brought into correspondence with a point
is brought into correspondence with a point 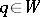 . Moreover, two non-intersecting neighbourhoods of the points
. Moreover, two non-intersecting neighbourhoods of the points 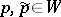 are situated over each neighbourhood of an interior point
are situated over each neighbourhood of an interior point 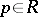 . If
. If  is a local uniformizing parameter in a neighbourhood of the interior point
is a local uniformizing parameter in a neighbourhood of the interior point 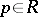 , it will also be a local uniformizing parameter in a
, it will also be a local uniformizing parameter in a 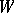 -neighbourhood of one out of the two conjugate points of
-neighbourhood of one out of the two conjugate points of 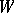 lying over
lying over 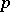 , say in a
, say in a 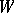 -neighbourhood of the point
-neighbourhood of the point 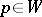 ; then, in a
; then, in a 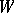 -neighbourhood of the conjugate point
-neighbourhood of the conjugate point 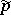 , the complex conjugate
, the complex conjugate  of the variable
of the variable  will be a local uniformizing parameter. If
will be a local uniformizing parameter. If  is a local uniformizing parameter at a point
is a local uniformizing parameter at a point 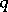 of the boundary of
of the boundary of 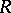 , then the variable which is equal to
, then the variable which is equal to  on one sheet of
on one sheet of 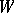 and to
and to  on the other will be a local uniformizing parameter at the point
on the other will be a local uniformizing parameter at the point 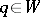 lying over it.
lying over it.
In the case of a compact orientable Riemann surface 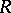 , the double simply consists of two compact orientable Riemann surfaces, and its study is accordingly of no interest. In all other cases the double of the Riemann surface is a compact orientable Riemann surface. This fact permits one to simplify the study of certain problems in the theory of functions on
, the double simply consists of two compact orientable Riemann surfaces, and its study is accordingly of no interest. In all other cases the double of the Riemann surface is a compact orientable Riemann surface. This fact permits one to simplify the study of certain problems in the theory of functions on 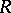 by reducing them to the study of functions on
by reducing them to the study of functions on 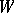 . The genus (cf. Genus of a surface) of
. The genus (cf. Genus of a surface) of 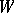 is
is 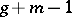 , where
, where 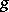 is the genus of
is the genus of 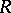 and
and 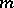 is the number of components of the boundary of
is the number of components of the boundary of 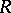 , which are assumed to be non-degenerate. For instance, the double of a simply-connected plane domain is a sphere, while the double of an
, which are assumed to be non-degenerate. For instance, the double of a simply-connected plane domain is a sphere, while the double of an 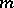 -connected plane domain is a sphere with
-connected plane domain is a sphere with  handles.
handles.
Analytic differentials on a Riemann surface (cf. Differential on a Riemann surface) 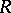 are analytic differentials on the double
are analytic differentials on the double 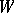 characterized by the fact that they assume conjugate values at conjugate points of
characterized by the fact that they assume conjugate values at conjugate points of 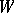 and take real values at the points
and take real values at the points 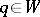 lying over points of the boundary of
lying over points of the boundary of 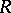 .
.
References
| [1] | M. Schiffer, D.C. Spencer, "Functionals of finite Riemann surfaces" , Princeton Univ. Press (1954) |
| [2] | E. Picard, "Traité d'analyse" , 2 , Gauthier-Villars (1926) |
Comments
The process of constructing the double of a Riemann surface is called duplication. This process can be applied to any connected two-dimensional manifold 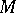 with boundary (cf. Boundary (of a manifold)) to yield a regular imbedding of
with boundary (cf. Boundary (of a manifold)) to yield a regular imbedding of 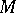 in a connected two-dimensional manifold (cf. [a1], § 13.H).
in a connected two-dimensional manifold (cf. [a1], § 13.H).
References
| [a1] | L.V. Ahlfors, L. Sario, "Riemann surfaces" , Princeton Univ. Press (1974) |
| [a2] | H.M. Farkas, I. Kra, "Riemann surfaces" , Springer (1980) |
| [a3] | G. Springer, "Introduction to Riemann surfaces" , Addison-Wesley (1957) pp. Chapt.10 |
Double of a Riemann surface. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Double_of_a_Riemann_surface&oldid=17837