Wall invariant

An element of the Wall group, representing the obstruction to the surgery of a bordism to a simple homotopy equivalence.

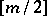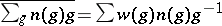Let be a finite Poincaré complex, a fibre bundle over and a bordism class, where is the formal dimension of and has degree 1. This mapping can always be represented by an -connected mapping using a finite sequence of surgeries. Let be a group ring and let be the involution on given by the formula , where is defined by the first Stiefel–Whitney class of . Put

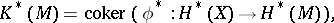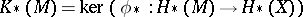(coefficients in 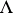). The involution is an anti-isomorphism and there is defined the Wall group .

Suppose now that . Then in the stable free -module 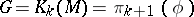one can choose a basis, and Poincaré duality induces a simple isomorphism , where is a -form. One therefore obtains the class .

Suppose next that . One can choose generators in so that they represent the imbeddings , with non-intersecting images, and these images are connected by paths with a base point. Put 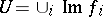, 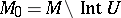. Since , one may replace by a homotopy and suppose that . Because is a Poincaré complex, one can replace by a complex with a unique 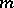-cell, i.e. one has a Poincaré pair and . By the choice of a suitable cellular approximation one obtains a mapping for the Poincaré triad of degree 1: . Consequently one has the diagram of exact sequences:

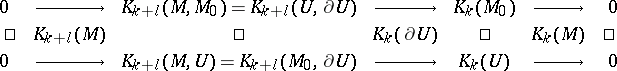Moreover, one has a non-degenerate pairing 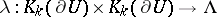, where is a quadratic -form while and 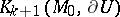define its Lagrange planes 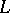and . Then .

The elements defined above are called the Wall invariants. An important property is the independence of on the choices in the construction and the equivalence of the equation to the representability of the class as a simple homotopy equivalence, cf. [1].

References

[1]	C.T.C. Wall, "Surgery on compact manifolds" , Acad. Press (1970)
[2]	A.A. Ranicki, "The algebraic theory of surgery I" Proc. London Math. Soc. , 40 : 1 (1980) pp. 87–192
[3]	S.P. Novikov, "Algebraic construction and properties of Hermitian analogs of -theory over rings with involution from the viewpoint of Hamiltonian formalism. Applications to differential topology and the theory of characteristic classes I" Math. USSR Izv. , 4 : 2 (1970) pp. 257–292 Izv. Akad. Nauk SSSR Ser. Mat. , 34 : 2 (1970) pp. 253–288