Inessential mapping
homotopically-trivial mapping
A continuous mapping 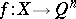 of a topological space
of a topological space 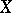 into the
into the  -dimensional ball
-dimensional ball  such that there is a continuous mapping
such that there is a continuous mapping 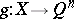 that coincides with
that coincides with 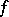 on the inverse image
on the inverse image 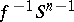 of the boundary
of the boundary 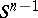 of
of 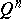 and takes
and takes 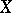 into
into 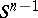 (that is,
(that is, 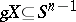 ). When
). When 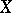 is a normal Hausdorff space, then
is a normal Hausdorff space, then 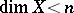 if and only if every continuous mapping
if and only if every continuous mapping 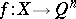 ,
, 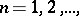 is inessential (Aleksandrov's theorem).
is inessential (Aleksandrov's theorem).
A continuous mapping of a topological space into the  -dimensional sphere is called inessential if it is homotopic to the constant mapping.
-dimensional sphere is called inessential if it is homotopic to the constant mapping.
Comments
The term "homotopically-trivial mapping" is not used in the context  . See [a1], [a2] and [a3].
. See [a1], [a2] and [a3].
Generally, a mapping which is homotopic to a constant mapping is called nullhomotopic or homotopically trivial; in [a3] they are called inessential. See also Essential mapping.
References
| [a1] | P.S. Aleksandrov, "Topologie" , 1 , Springer (1974) |
| [a2] | R. Engelking, "Dimension theory" , PWN (1977) |
| [a3] | W. Hurevicz, G. Wallman, "Dimension theory" , Princeton Univ. Press (1948) |
Inessential mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Inessential_mapping&oldid=17383