Defect(2)
ramification deficiency
An invariant of finite extensions  of fields equipped with a valuation (cf. also Extension of a field). If a valuation
of fields equipped with a valuation (cf. also Extension of a field). If a valuation  on
on  is the unique extension of a valuation
is the unique extension of a valuation  on
on  , then the defect (or ramification deficiency)
, then the defect (or ramification deficiency)  is defined by the formula
is defined by the formula  , where
, where  is the degree of
is the degree of  (i.e., the dimension of
(i.e., the dimension of  as a
as a 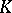 -vector space),
-vector space), 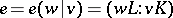 is the ramification index and
is the ramification index and 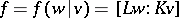 is the inertia degree. Here,
is the inertia degree. Here,  denote the respective value groups and
denote the respective value groups and  the respective residue fields. If
the respective residue fields. If  admits several extensions to
admits several extensions to  , the defect
, the defect  can be defined by
can be defined by  , where
, where 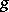 is the number of distinct extensions, provided that
is the number of distinct extensions, provided that 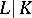 is normal (since in that case the ramification index and the inertia degree are the same for all extensions; cf. also Normal extension).
is normal (since in that case the ramification index and the inertia degree are the same for all extensions; cf. also Normal extension).
In the above cases,  ,
, 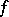 ,
, 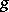 are divisors of
are divisors of 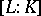 . The defect
. The defect  is either equal to
is either equal to 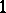 or is a power of the characteristic
or is a power of the characteristic 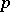 of
of 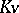 if
if  ; otherwise, it is always equal to
; otherwise, it is always equal to  (this is the Ostrowski lemma, cf. Ramification theory of valued fields).
(this is the Ostrowski lemma, cf. Ramification theory of valued fields).
Henselian defect.
To avoid considering several valuations and to have a defect available for arbitrary finite extensions, one can pass to a Henselization  of
of  and a Henselization
and a Henselization 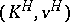 of
of 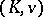 inside
inside 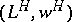 (cf. Henselization of a valued field). The Henselian defect
(cf. Henselization of a valued field). The Henselian defect  is then defined to be the defect of
is then defined to be the defect of 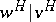 (by the definition of the Henselization,
(by the definition of the Henselization,  is the unique extension of
is the unique extension of  ). In the above cases,
). In the above cases, 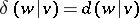 .
.
Defectless fields.
A field  with a valuation
with a valuation  is called a defectless field if
is called a defectless field if  for every finite normal extension. This holds if and only if the Henselian defect is equal to
for every finite normal extension. This holds if and only if the Henselian defect is equal to 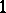 for every finite extension. It follows that
for every finite extension. It follows that 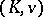 is a defectless field if and only some Henselization of
is a defectless field if and only some Henselization of 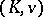 is (or equivalently, all Henselizations are).
is (or equivalently, all Henselizations are).
It follows from the Ostrowski lemma that all valued fields with residue field of characteristic  are defectless fields. Also, valued fields of characteristic
are defectless fields. Also, valued fields of characteristic  with value group isomorphic to
with value group isomorphic to 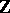 are defectless. Combining both facts, it is shown that finitely ramified fields, and hence also fields with
are defectless. Combining both facts, it is shown that finitely ramified fields, and hence also fields with  -valuations (see
-valuations (see  -adically closed field), are defectless.
-adically closed field), are defectless.
If a valued field does not admit any non-trivial immediate extension (cf. also Valuation), then it is called a maximal valued field. Fields of formal Laurent series with their canonical valuations are maximal. Every maximal valued field is defectless.
Fundamental inequality.
If 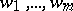 are all extensions of
are all extensions of  from
from  to
to  , then one has the fundamental inequality
, then one has the fundamental inequality
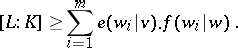 |
This is an equality for every finite  if and only if
if and only if  is defectless. Also, in general it can be written as an equality. For this, choose Henselizations
is defectless. Also, in general it can be written as an equality. For this, choose Henselizations 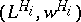 of
of  and
and 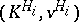 of
of 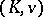 inside
inside  . It is known that
. It is known that
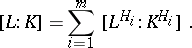 |
Further,
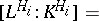 |
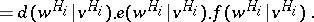 |
Since Henselizations are immediate extensions, 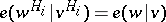 and
and 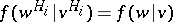 . By definition,
. By definition, 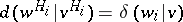 . Hence,
. Hence,
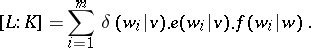 |
Several other notions of defects were introduced. For a detailed theory of the defect, see [a1]. See also Valued function field.
References
| [a1] | F.-V. Kuhlmann, "Valuation theory of fields, abelian groups and modules" , Algebra, Logic and Applications , Gordon&Breach (to appear) |
Defect(2). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Defect(2)&oldid=17106