Interval

See Interval and segment.

A space-time interval is a quantity characterizing the relation between two events separated by a spatial distance and a time duration. In special relativity theory the square of an interval is

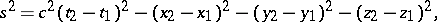where is the velocity of light, are the space coordinates and are the corresponding points in time (for more details, see Minkowski space).

In general relativity theory one considers the interval between two infinitesimally-close events:

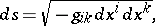where is the infinitesimal difference of the space-time coordinates of these events and is the metric tensor.

Comments

A space-time interval with is called a time-like space-time interval, and one with is called a space-like space-time interval.

References

[a1]	D.F. Lawden, "An introduction to tensor calculus and relativity" , Methuen (1962)
[a2]	R.K. Sachs, H. Wu, "General relativity for mathematicians" , Springer (1977)
[a3]	E. Tocaci, "Relativistic mechanics, time, and inertia" , Reidel (1985) pp. Sect. A.II.1.4