Hadwiger hypothesis

Hadwiger conjecture

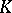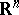A problem in combinatorial geometry on the covering of a convex body by figures of a special form, which was put forth by H. Hadwiger in [1]. Let be a convex body in the -dimensional Euclidean space , and let 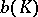the minimal number of bodies homothetic to with homothety coefficient 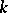, , 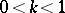that are sufficient to cover 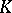. The Hadwiger conjecture consists in the following: Any bounded set 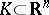satisfies the inequality

(*)

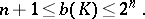Here the equality characterizes a parallelepiped (see [1]). The Hadwiger conjecture has been proved 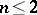for ; for 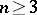there are (1988) only partial results. For example, for any -dimensional bounded polyhedron 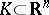in which any two vertices belong to two distinct parallel supporting hyperplanes to 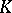the inequality (*) holds. Here coincides with the number of vertices of 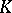, but in the set of such polyhedra the equality 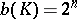has been verified only for parallelepipeds. This result is connected with the solution of the Erdös problem on the number of points in 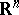any three of which form a triangle that is not obtuse angled. The Hadwiger conjecture is also connected with covering; decomposition and the illumination problem. For example, the Hadwiger conjecture can be regarded as a generalization of the Borsuk problem on the decomposition of a set into parts of smaller diameter, when is replaced by a Minkowski space. For an unbounded set 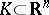the number 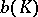is either equal to 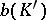, where 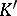is a convex bounded body of lower dimension, or is 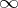. For example, for 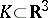the number 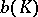can only take one of the values (see [2]).

References

[1]	H. Hadwiger, "Ueber Treffanzahlen bei translationsgleichen Eikörpern" Arch. Math. (Basel) , 8 (1957) pp. 212–213
[2]	V.G. Boltyanskii, P.S. Soltan, "The combinatorial geometry of various classes of convex sets" , Kishinev (1978) (In Russian)

Comments

For bounded centrally-symmetric bodies Hadwiger's conjecture holds, see [a1].

See also Geometry of numbers and the standard work [a4].

References

[a1]	M. Lassak, "Solution of Hadwiger's covering problem for centrally symmetric convex bodies in 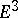" J. London Math. Soc. (2) , 30 (1984) pp. 501–511
[a2]	L. Danzer, B. Grünbaum, V.L. Klee, "Helly's theorem and its relatives" V.L. Klee (ed.) , Convexity , Proc. Symp. Pure Math. , 7 , Amer. Math. Soc. (1963) pp. 101–180
[a3]	H. Hadwiger, H. Debrunner, "Kombinatorische Geometrie in der Ebene" L'Enseign. Math. , 2 (1959)
[a4]	P.M. Gruber, C.G. Lekkerkerker, "Geometry of numbers" , North-Holland (1987) pp. Sect. (iv) (Updated reprint)